24
ENGINEER AND MACHINIST’S DRAWING-BOOK.
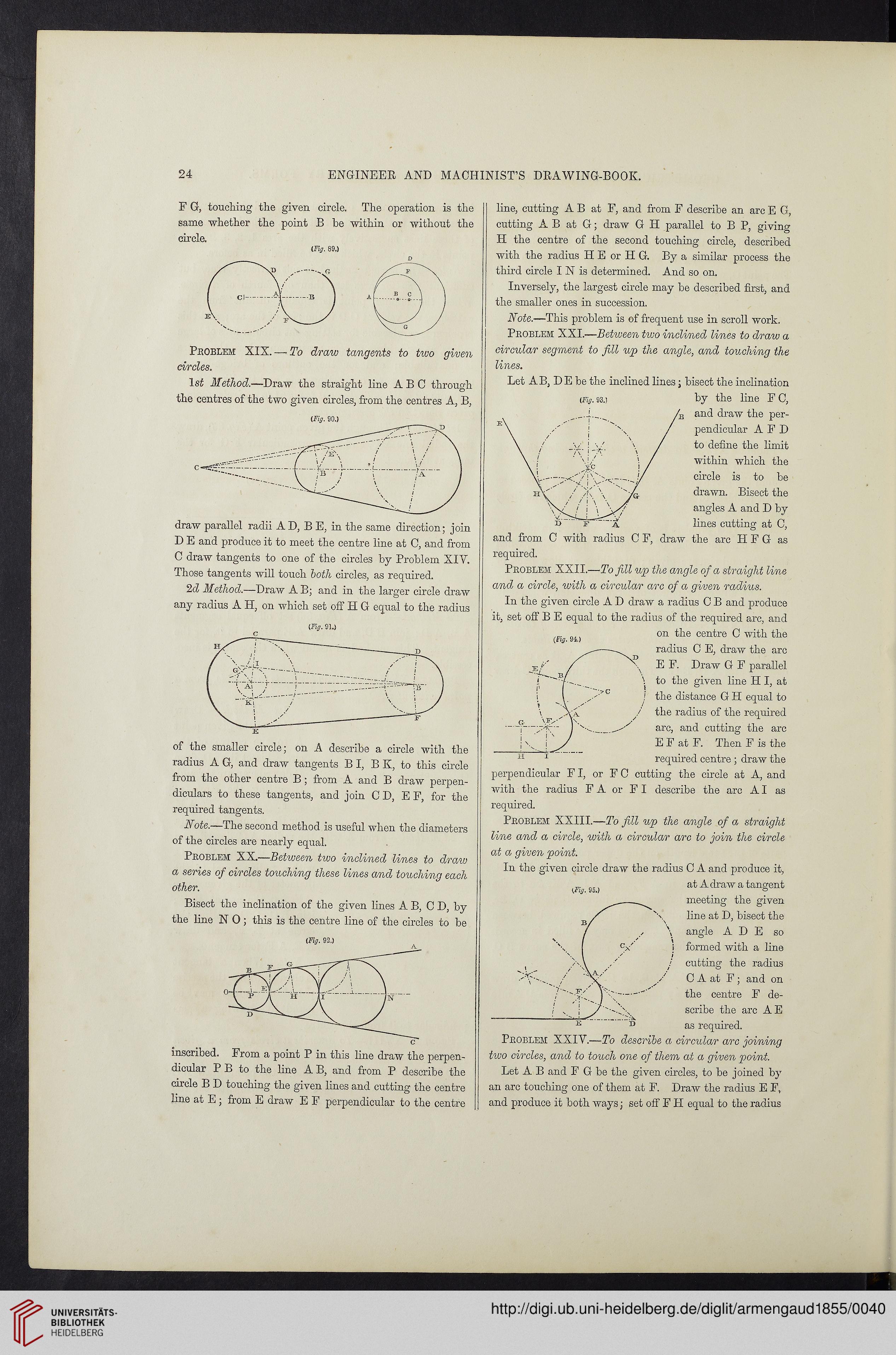
F G, touching the given circle. The operation is the
same whether the point B he within or without the
circle.
(Fig. 89.)
Problem XIX. — To draw tangents to two given
circles.
lsi Method.—Draw the straight line ABC through
the centres of the two given circles, from the centres A, B,
(Fig. 90.)
draw parallel radii AD, B E, in the same direction; join
D E and produce it to meet the centre line at C, and from
C draw tangents to one of the circles by Problem XIY.
Those tangents will touch both circles, as required.
2d Method.—Draw A B; and in the larger circle draw
any radius A H, on which set off H G equal to the radius
(Fig. 91.)
c
of the smaller circle; on A describe a circle with the
radius A G, and draw tangents B I, B K, to this circle
from the other centre B; from A and B draw perpen-
diculars to these tangents, and join CD, E F, for the
required tangents.
Note.—The second method is useful when the diameters
of the circles are nearly equal.
Problem XX.—Between two inclined lines to draiu
a series of circles touching these lines and touching each
other.
Bisect the inclination of the given lines A B, CD, by
the line N 0; this is the centre line of the circles to be
(Tig. 92.)
inscribed. From a point P in this line draw the perpen-
dicular P B to the line A B, and from P describe the
circle B D touching the given lines and cutting the centre
line at E ; from E draw E F perpendicular to the centre
(Fig. 93.)
line, cutting A B at F, and from F describe an arc E G,
cutting A B at G; draw G H parallel to B P, giving
H the centre of the second touching circle, described
with the radius H E or H G. By a similar process the
third circle I N is determined. And so on.
Inversely, the largest circle may be described first, and
the smaller ones in succession.
Note.—This problem is of frequent use in scroll work.
Problem XXI.—Between two inclined lines to draw a
circular segment to fill up the angle, and touching the
lines.
Let AB, D E be the inclined lines; bisect the inclination
by the line F C,
and draw the per-
pendicular A F D
to define the limit
within which the
circle is to be
drawn. Bisect the
angles A and D by
f a lines cutting at C,
and from C with radius C F, draw the arc H F G as
required.
Problem XXII.—To fill up the angle of a straight line
and a circle, with a circular arc of a given radius.
In the given circle A D draw a radius C B and produce
it, set off B E equal to the radius of the required arc, and
on the centre C with the
{Fig. 94.)
radius C E, draw the arc
E F. Draw G F parallel
to the given line H I, at
the distance G PI equal to
the radius of the required
arc, and cutting the arc
E F at F. Then F is the
required centre ; draw the
perpendicular FI, or F C cutting the circle at A, and
with the radius F A or FI describe the arc AI as
required.
Problem XXIII.—To fill up the angle of a straight
line and a circle, with a circular arc to join the circle
at a given point.
In the given circle draw the radius C A and produce it,
at A draw a tangent
mg. 95.) _ °
meeting the given
line at D, bisect the
angle A D E so
formed with a line
cutting the radius
C A at F ; and on
the centre F de-
scribe the arc AE
as required.
Problem XXIV.—To describe a circular arc joining
two circles, and to touch one of them at a given point.
Let A B and F G be the given circles, to be joined by
an arc touching one of them at F. Draw the radius E F,
and produce it both ways; set off F H equal to the radius
ENGINEER AND MACHINIST’S DRAWING-BOOK.
F G, touching the given circle. The operation is the
same whether the point B he within or without the
circle.
(Fig. 89.)
Problem XIX. — To draw tangents to two given
circles.
lsi Method.—Draw the straight line ABC through
the centres of the two given circles, from the centres A, B,
(Fig. 90.)
draw parallel radii AD, B E, in the same direction; join
D E and produce it to meet the centre line at C, and from
C draw tangents to one of the circles by Problem XIY.
Those tangents will touch both circles, as required.
2d Method.—Draw A B; and in the larger circle draw
any radius A H, on which set off H G equal to the radius
(Fig. 91.)
c
of the smaller circle; on A describe a circle with the
radius A G, and draw tangents B I, B K, to this circle
from the other centre B; from A and B draw perpen-
diculars to these tangents, and join CD, E F, for the
required tangents.
Note.—The second method is useful when the diameters
of the circles are nearly equal.
Problem XX.—Between two inclined lines to draiu
a series of circles touching these lines and touching each
other.
Bisect the inclination of the given lines A B, CD, by
the line N 0; this is the centre line of the circles to be
(Tig. 92.)
inscribed. From a point P in this line draw the perpen-
dicular P B to the line A B, and from P describe the
circle B D touching the given lines and cutting the centre
line at E ; from E draw E F perpendicular to the centre
(Fig. 93.)
line, cutting A B at F, and from F describe an arc E G,
cutting A B at G; draw G H parallel to B P, giving
H the centre of the second touching circle, described
with the radius H E or H G. By a similar process the
third circle I N is determined. And so on.
Inversely, the largest circle may be described first, and
the smaller ones in succession.
Note.—This problem is of frequent use in scroll work.
Problem XXI.—Between two inclined lines to draw a
circular segment to fill up the angle, and touching the
lines.
Let AB, D E be the inclined lines; bisect the inclination
by the line F C,
and draw the per-
pendicular A F D
to define the limit
within which the
circle is to be
drawn. Bisect the
angles A and D by
f a lines cutting at C,
and from C with radius C F, draw the arc H F G as
required.
Problem XXII.—To fill up the angle of a straight line
and a circle, with a circular arc of a given radius.
In the given circle A D draw a radius C B and produce
it, set off B E equal to the radius of the required arc, and
on the centre C with the
{Fig. 94.)
radius C E, draw the arc
E F. Draw G F parallel
to the given line H I, at
the distance G PI equal to
the radius of the required
arc, and cutting the arc
E F at F. Then F is the
required centre ; draw the
perpendicular FI, or F C cutting the circle at A, and
with the radius F A or FI describe the arc AI as
required.
Problem XXIII.—To fill up the angle of a straight
line and a circle, with a circular arc to join the circle
at a given point.
In the given circle draw the radius C A and produce it,
at A draw a tangent
mg. 95.) _ °
meeting the given
line at D, bisect the
angle A D E so
formed with a line
cutting the radius
C A at F ; and on
the centre F de-
scribe the arc AE
as required.
Problem XXIV.—To describe a circular arc joining
two circles, and to touch one of them at a given point.
Let A B and F G be the given circles, to be joined by
an arc touching one of them at F. Draw the radius E F,
and produce it both ways; set off F H equal to the radius