GEOMETRICAL CONSTRUCTIONS—DRAWING OF ELEMENTARY FORMS.
25
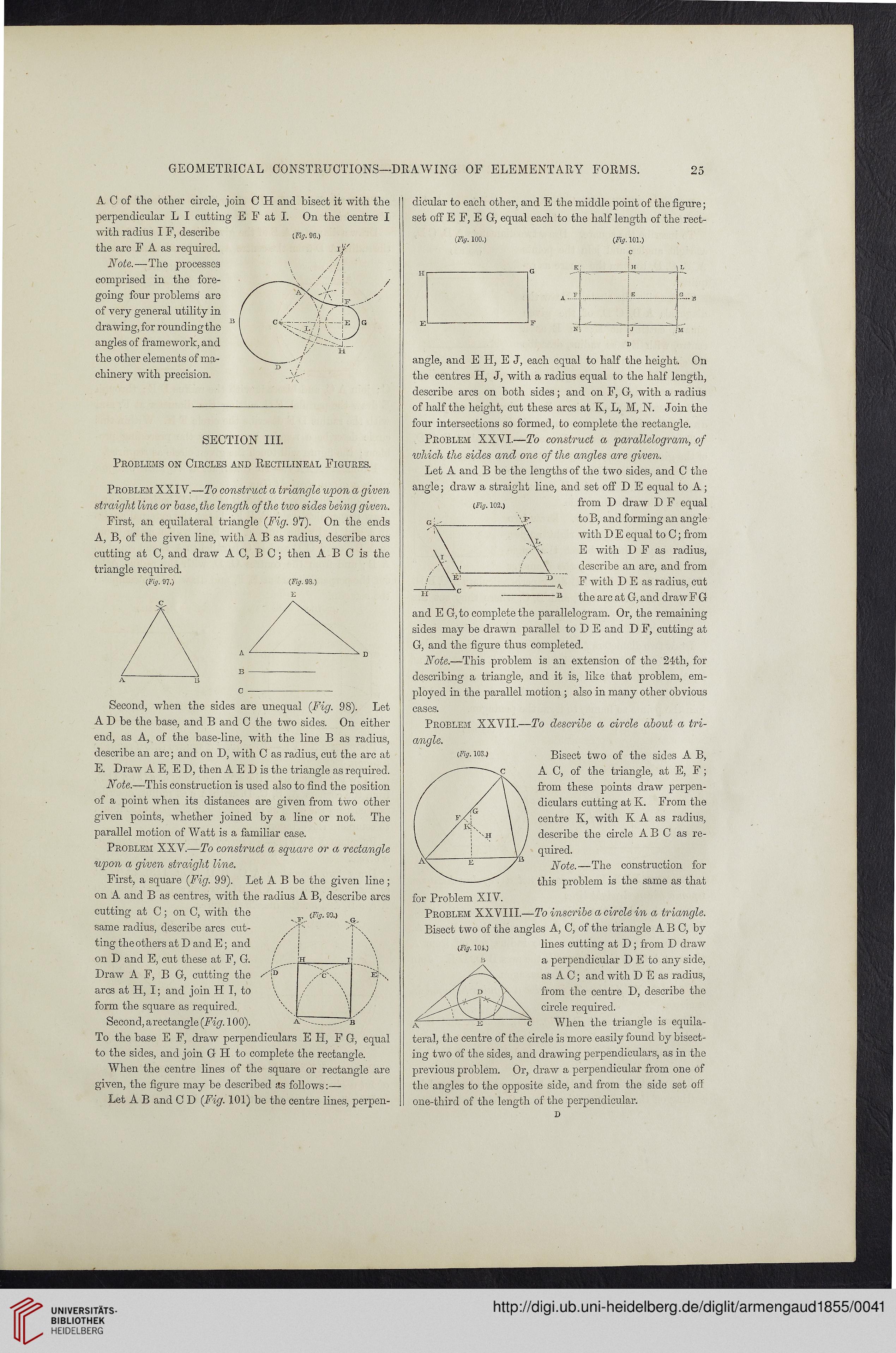
A C of tlie other circle, join C H and bisect it with the
perpendicular L I cutting E F at I. On the centre I
with radius I F, describe
the arc F A as required.
Note.—The processes
comprised in the fore-
going four problems are
of very general utility in
drawing, for rounding the
angles of framework, and
the other elements of ma-
chinery with precision.
SECTION III.
Problems on Circles and Rectilineal Figures.
Problem XXIY.—To construct a triangle upon a given
straight line or base, the length of the two sides being given.
First, an equilateral triangle (Fig. 97). On the ends
A, B, of the given line, with A B as radius, describe arcs
cutting at C, and draw AC, B C; then A B C is the
triangle required.
(Fig. 97.) (Fig. 9S.)
B
A Ii
C —---
Second, when the sides are unequal (Fig. 98). Let
A D be the base, and B and C the two sides. On either
end, as A, of the base-line, with the line B as radius,
describe an arc; and on D, with C as radius, cut the arc at
E. Draw A E, E D, then A E D is the triangle as required.
Note.—This construction is used also to find the position
of a point when its distances are given from two other
given points, whether joined by a line or not. The
parallel motion of Watt is a familiar case.
Problem XXY.—To construct a square or a rectangle
upon a given straight line.
First, a square (Fig. 99). Let A B be the given line;
on A and B as centres, with the radius A B, describe arcs
cutting at C ; on C, with the
same radius, describe arcs cut-
ting the others at D and E; and
on D and E, cut these at F, G.
Draw A F, B G, cutting the
arcs at H, I; and join H I, to
form the square as required.
Second, a rectangle (Fig. 100).
To the base E F, draw perpendiculars E IT, F G, equal
to the sides, and join G PI to complete the rectangle.
When the centre lines of the square or rectangle are
given, the figure may be described as follows:—
Let A B and C D (Fig. 101) be the centre lines, perpen-
dicular to each other, and E the middle point of the figure;
set off E F, E G, equal each to the half length of the rect-
(Fig. 100.) (Fig. 101.)
c
angle, and E H, E J, each equal to half the height. On
the centres H, J, with a radius equal to the half length,
describe arcs on both sides; and on F, G, with a radius
of half the height, cut these arcs at K, L, M, N. Join the
four intersections so formed, to complete the rectangle.
Problem XXYI.—To construct a parallelogram, of
vjhich the sides and one of the angles are given.
Let A and B be the lengths of the two sides, and C the
angle; draw a straight line, and set off D E equal to A;
from D draw D F equal
to B, and forming an angle
with D E equal to C; from
E with D F as radius,
describe an arc, and from
F with D E as radius, cut
the arc at G, and drawF G
and E G, to complete the parallelogram. Or, the remaining
sides may be drawn parallel to D E and D F, cutting at
G, and the figure thus completed.
Note.—This problem is an extension of the 24th, for
describing a triangle, and it is, like that problem, em-
ployed in the parallel motion ; also in many other obvious
cases.
Problem XXYII.—To describe a circle about a tri-
(Fig. 103.)
angle.
(Fig. 103.)
Bisect two of the sides A B,
A C, of the triangle, at E, F;
from these points draw perpen-
diculars cutting at K. From the
centre K, with K A as radius,
describe the circle A B C as re-
quired.
Note.—The construction for
this problem is the same as that
for Problem XIY.
Problem XXYIII.—To inscribe a circle in a triangle.
Bisect two of the angles A, C, of the triangle A B C, by
lines cutting at D; from D draw
a perpendicular D E to any side,
as A C; and with D E as radius,
from the centre D, describe the
circle required.
When the triangle is equila-
teral, the centre of the circle is more easily found by bisect-
ing two of the sides, and drawing perpendiculars, as in the
previous problem. Or, draw a perpendicular from one of
the angles to the opposite side, and from the side set off
one-third of the length of the perpendicular.
D
25
A C of tlie other circle, join C H and bisect it with the
perpendicular L I cutting E F at I. On the centre I
with radius I F, describe
the arc F A as required.
Note.—The processes
comprised in the fore-
going four problems are
of very general utility in
drawing, for rounding the
angles of framework, and
the other elements of ma-
chinery with precision.
SECTION III.
Problems on Circles and Rectilineal Figures.
Problem XXIY.—To construct a triangle upon a given
straight line or base, the length of the two sides being given.
First, an equilateral triangle (Fig. 97). On the ends
A, B, of the given line, with A B as radius, describe arcs
cutting at C, and draw AC, B C; then A B C is the
triangle required.
(Fig. 97.) (Fig. 9S.)
B
A Ii
C —---
Second, when the sides are unequal (Fig. 98). Let
A D be the base, and B and C the two sides. On either
end, as A, of the base-line, with the line B as radius,
describe an arc; and on D, with C as radius, cut the arc at
E. Draw A E, E D, then A E D is the triangle as required.
Note.—This construction is used also to find the position
of a point when its distances are given from two other
given points, whether joined by a line or not. The
parallel motion of Watt is a familiar case.
Problem XXY.—To construct a square or a rectangle
upon a given straight line.
First, a square (Fig. 99). Let A B be the given line;
on A and B as centres, with the radius A B, describe arcs
cutting at C ; on C, with the
same radius, describe arcs cut-
ting the others at D and E; and
on D and E, cut these at F, G.
Draw A F, B G, cutting the
arcs at H, I; and join H I, to
form the square as required.
Second, a rectangle (Fig. 100).
To the base E F, draw perpendiculars E IT, F G, equal
to the sides, and join G PI to complete the rectangle.
When the centre lines of the square or rectangle are
given, the figure may be described as follows:—
Let A B and C D (Fig. 101) be the centre lines, perpen-
dicular to each other, and E the middle point of the figure;
set off E F, E G, equal each to the half length of the rect-
(Fig. 100.) (Fig. 101.)
c
angle, and E H, E J, each equal to half the height. On
the centres H, J, with a radius equal to the half length,
describe arcs on both sides; and on F, G, with a radius
of half the height, cut these arcs at K, L, M, N. Join the
four intersections so formed, to complete the rectangle.
Problem XXYI.—To construct a parallelogram, of
vjhich the sides and one of the angles are given.
Let A and B be the lengths of the two sides, and C the
angle; draw a straight line, and set off D E equal to A;
from D draw D F equal
to B, and forming an angle
with D E equal to C; from
E with D F as radius,
describe an arc, and from
F with D E as radius, cut
the arc at G, and drawF G
and E G, to complete the parallelogram. Or, the remaining
sides may be drawn parallel to D E and D F, cutting at
G, and the figure thus completed.
Note.—This problem is an extension of the 24th, for
describing a triangle, and it is, like that problem, em-
ployed in the parallel motion ; also in many other obvious
cases.
Problem XXYII.—To describe a circle about a tri-
(Fig. 103.)
angle.
(Fig. 103.)
Bisect two of the sides A B,
A C, of the triangle, at E, F;
from these points draw perpen-
diculars cutting at K. From the
centre K, with K A as radius,
describe the circle A B C as re-
quired.
Note.—The construction for
this problem is the same as that
for Problem XIY.
Problem XXYIII.—To inscribe a circle in a triangle.
Bisect two of the angles A, C, of the triangle A B C, by
lines cutting at D; from D draw
a perpendicular D E to any side,
as A C; and with D E as radius,
from the centre D, describe the
circle required.
When the triangle is equila-
teral, the centre of the circle is more easily found by bisect-
ing two of the sides, and drawing perpendiculars, as in the
previous problem. Or, draw a perpendicular from one of
the angles to the opposite side, and from the side set off
one-third of the length of the perpendicular.
D