26
ENGINEER AND MACHINIST’S DRAWING-BOOK.
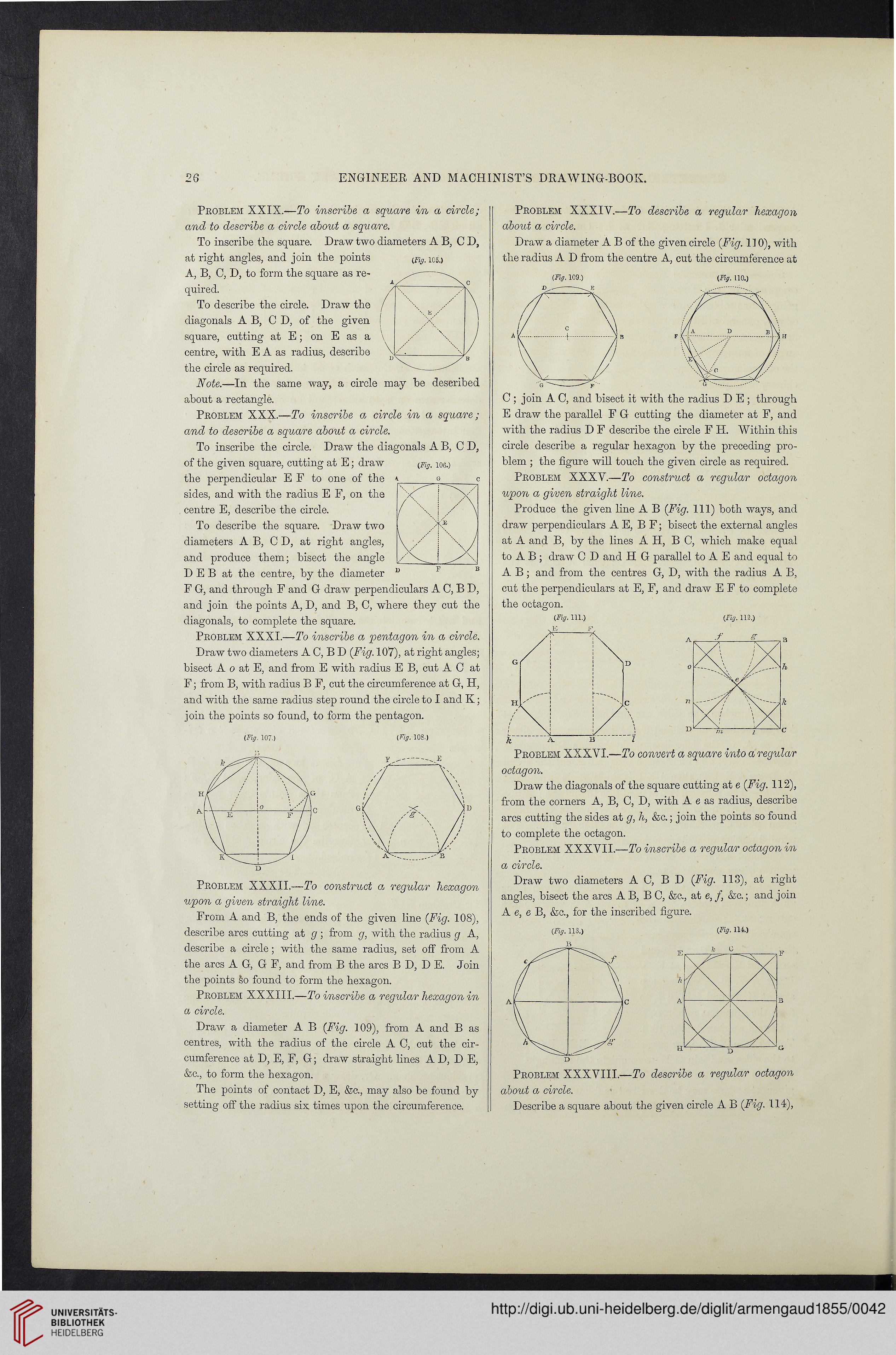
Problem XXIX.—To inscribe a square in a circle;
and to describe a circle about a square.
To inscribe the square. Draw two diameters A B, CD,
at right angles, and join the points ^g. 105.)
A, B, C, D, to form the square as re-
quired.
To describe the circle. Draw the
diagonals A B, CD, of the given
square, cutting at E; on E as a
centre, with E A as radius, describe
the circle as required.
Note.—In the same way, a circle may be described
about a rectangle.
Problem XXX.—To inscribe a circle in a square;
and to describe a square about a circle.
To inscribe the circle. Draw the diagonals AB, CD,
of the given square, cutting at E; draw (Fig. 100.)
the perpendicular E F to one of the
sides, and with the radius E F, on the
centre E, describe the circle.
To describe the square. Draw two
diameters A B, CD, at right angles,
and produce them; bisect the angle
D E B at the centre, by the diameter
F G, and through F and G draw perpendiculars A C, B D,
and join the points A, D, and B, C, where they cut the
diagonals, to complete the square.
Problem XXXI.—To inscribe a pentagon in a circle.
Draw two diameters A C, B D (Fig. 107), at right angles;
bisect A 0 at E, and from E with radius E B, cut A C at
F; from B, with radius B F, cut the circumference at G, H,
and with the same radius step round the circle to I and K;
join the points so found, to form the pentagon.
(Fig. 107.) (Fig. 108.)
Problem XXXII.—To construct a regular hexagon
upon a given straight line.
From A and B, the ends of the given line {Fig. 108),
describe arcs cutting at g; from g, with the radius g A,
describe a circle; with the same radius, set off from A
the arcs A G, G F, and from B the arcs B D, D E. Join
the points ho found to form the hexagon.
Problem XXXIII.—To inscribe a regular hexagon in
a circle.
Draw a diameter A B {Fig. 109), from A and B as
centres, with the radius of the circle A C, cut the cir-
cumference at D, E, F, G; draw straight lines A D, D E,
&c., to form the hexagon.
The points of contact D, E, &c., may also be found by
setting off the radius six times upon the circumference.
Problem XXXIV.—To describe a regular hexagon
about a circle.
Draw a diameter A B of the given circle {Fig. 110), with
the radius A D from the centre A, cut the circumference at
(Fig. 109.) (Fig. 110.)
C ; join A C, and bisect it with the radius D E ; through
E draw the parallel F G cutting the diameter at F, and
with the radius D F describe the circle F H. Within this
circle describe a regular hexagon by the preceding pro-
blem ; the figure will touch the given circle as required.
Problem XXXV.—To construct a regular octagon
upon a given straight line.
Produce the given line A B {Fig. Ill) both ways, and
draw perpendiculars A E, B F; bisect the external angles
at A and B, by the lines AH, B C, which make equal
to A B ; draw C D and H G parallel to A E and equal to
A B; and from the centres G, D, with the radius A B,
cut the perpendiculars at E, F, and draw E F to complete
the octagon.
(Fig. 111.) (Fig. 112.)
Problem XXXVI.—To convert a square into a regular
octagon.
Draw the diagonals of the square cutting at e {Fig. 112),
from the corners A, B, C, D, with A e as radius, describe
i arcs cutting the sides at g, h, &c.; join the points so found
I to complete the octagon.
Problem XXXVII.—To inscribe a regular octagon in
a circle.
Draw two diameters A C, B D {Fig. 113), at right
angles, bisect the arcs A B, B C, &c., at e, /, &c.; and join
A e, e B, &c., for the inscribed figure.
(Fig. 113.) (Fig. 114)
D
Problem XXXVIII.—To describe a regular octagon
about a circle.
Describe a square about the given circle A B {Fig. 114),
ENGINEER AND MACHINIST’S DRAWING-BOOK.
Problem XXIX.—To inscribe a square in a circle;
and to describe a circle about a square.
To inscribe the square. Draw two diameters A B, CD,
at right angles, and join the points ^g. 105.)
A, B, C, D, to form the square as re-
quired.
To describe the circle. Draw the
diagonals A B, CD, of the given
square, cutting at E; on E as a
centre, with E A as radius, describe
the circle as required.
Note.—In the same way, a circle may be described
about a rectangle.
Problem XXX.—To inscribe a circle in a square;
and to describe a square about a circle.
To inscribe the circle. Draw the diagonals AB, CD,
of the given square, cutting at E; draw (Fig. 100.)
the perpendicular E F to one of the
sides, and with the radius E F, on the
centre E, describe the circle.
To describe the square. Draw two
diameters A B, CD, at right angles,
and produce them; bisect the angle
D E B at the centre, by the diameter
F G, and through F and G draw perpendiculars A C, B D,
and join the points A, D, and B, C, where they cut the
diagonals, to complete the square.
Problem XXXI.—To inscribe a pentagon in a circle.
Draw two diameters A C, B D (Fig. 107), at right angles;
bisect A 0 at E, and from E with radius E B, cut A C at
F; from B, with radius B F, cut the circumference at G, H,
and with the same radius step round the circle to I and K;
join the points so found, to form the pentagon.
(Fig. 107.) (Fig. 108.)
Problem XXXII.—To construct a regular hexagon
upon a given straight line.
From A and B, the ends of the given line {Fig. 108),
describe arcs cutting at g; from g, with the radius g A,
describe a circle; with the same radius, set off from A
the arcs A G, G F, and from B the arcs B D, D E. Join
the points ho found to form the hexagon.
Problem XXXIII.—To inscribe a regular hexagon in
a circle.
Draw a diameter A B {Fig. 109), from A and B as
centres, with the radius of the circle A C, cut the cir-
cumference at D, E, F, G; draw straight lines A D, D E,
&c., to form the hexagon.
The points of contact D, E, &c., may also be found by
setting off the radius six times upon the circumference.
Problem XXXIV.—To describe a regular hexagon
about a circle.
Draw a diameter A B of the given circle {Fig. 110), with
the radius A D from the centre A, cut the circumference at
(Fig. 109.) (Fig. 110.)
C ; join A C, and bisect it with the radius D E ; through
E draw the parallel F G cutting the diameter at F, and
with the radius D F describe the circle F H. Within this
circle describe a regular hexagon by the preceding pro-
blem ; the figure will touch the given circle as required.
Problem XXXV.—To construct a regular octagon
upon a given straight line.
Produce the given line A B {Fig. Ill) both ways, and
draw perpendiculars A E, B F; bisect the external angles
at A and B, by the lines AH, B C, which make equal
to A B ; draw C D and H G parallel to A E and equal to
A B; and from the centres G, D, with the radius A B,
cut the perpendiculars at E, F, and draw E F to complete
the octagon.
(Fig. 111.) (Fig. 112.)
Problem XXXVI.—To convert a square into a regular
octagon.
Draw the diagonals of the square cutting at e {Fig. 112),
from the corners A, B, C, D, with A e as radius, describe
i arcs cutting the sides at g, h, &c.; join the points so found
I to complete the octagon.
Problem XXXVII.—To inscribe a regular octagon in
a circle.
Draw two diameters A C, B D {Fig. 113), at right
angles, bisect the arcs A B, B C, &c., at e, /, &c.; and join
A e, e B, &c., for the inscribed figure.
(Fig. 113.) (Fig. 114)
D
Problem XXXVIII.—To describe a regular octagon
about a circle.
Describe a square about the given circle A B {Fig. 114),