GEOMETRICAL CONSTRUCTIONS—DRAWING OF ELEMENTARY FORMS.
31
off B E equal to the side B C of the rectangle ; bisect A E
at K, and describe a semicircle on A E ; draw the perpen-
dicular B H, cutting the circle at H, and on B H describe
the square B G required.
SECTION Y.
Problems on the Ellipse and the Parabola.
The Ellipse.
Definitions.—An ellipse (Fig. 141) is an oval-shaped
curve. The line A B that divides it into two equal and
symmetrical parts, is the transverse axis. The line D E,
perpendicular to the transverse at its centre point C, is
the conjugate axis. The points A, B, are the vertices of
the curve. The points F, and F', in the transverse axis,
are the foci of the curve, each being termed a focus ; and
they are so placed that their united distances from either
end of the conjugate axis, are equal together to the length
of the transverse axis. By the same rule, the distance of
each focus from the end of the conjugate axis, is equal to
the half-length of the transverse axis.
The ellipse has the characteristic property, that the
sum of the distances of any point in the curve from the
foci, is equal to the transverse axis ; so that the distances,
jointly, of all the points in the curve from the foci, are
equal.
Problem L.—To describe an ellipse, the length and
breadth, or the two axes, being given.
1st Method.—Bisect the transverse axis A B at C, and
through C draw the perpendicular D E, making C D and
C E each equal to half the conjugate diameter. On D as
a centre, with C A as radius, describe arcs cutting at
F, F', for the foci. Divide A C into a number of parts at
the points 1, 2, 3, &c. With radius A 1, on F and F' as
centres, describe arcs; and with radius B 1, on the same
centres, describe arcs intersecting the others as shown.
Repeat the operation for the other divisions of the trans-
verse axis. The series of intersections thus found will
be points in the curve, and they may be as numerously
found as is desirable ; after which a curve traced through
them will form the complete ellipse.
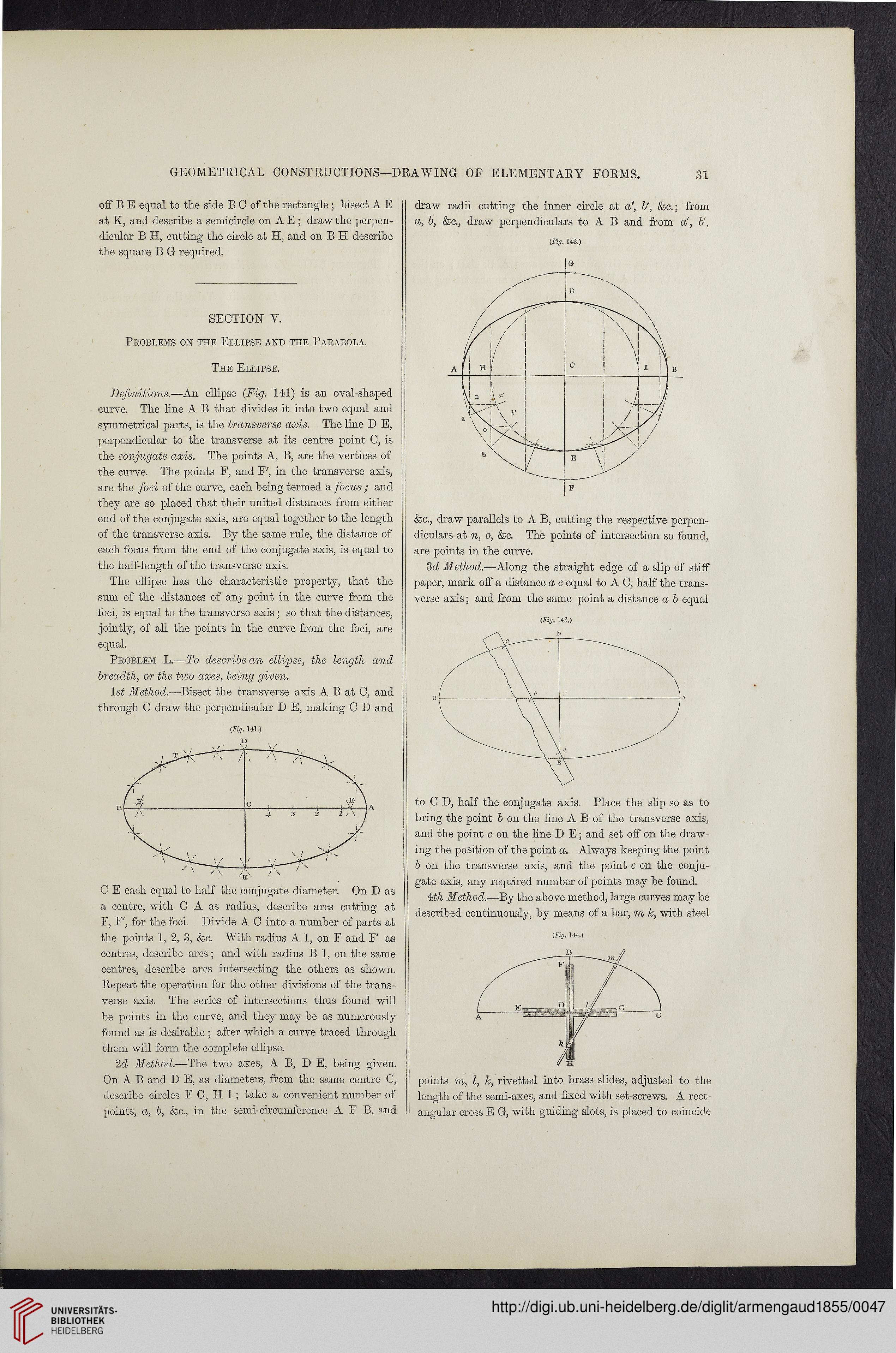
2d Method.—The two axes, A B, D E, being given.
On A B and D E, as diameters, from the same centre C,
describe circles F G, H I; take a convenient number of
points, a, b, &c., in the semi-circumference A F B, and
draw radii cutting the inner circle at a', b', &c.; from
a, b, &c., draw perpendiculars to A B and from a', b\
(.Fig. 140.)
&c., draw parallels to A B, cutting the respective perpen-
diculars at n, o, &c. The points of intersection so found,
are points in the curve.
3d Method.—Along the straight edge of a slip of stiff
paper, mark off a distance a c equal to A C, half the trans-
verse axis; and from the same point a distance a b equal
(Fig. 143.)
to C D, half the conjugate axis. Place the slip so as to
bring the point b on the line A B of the transverse axis,
and the point c on the line D E; and set off on the draw-
ing the position of the point a. Always keeping the point
b on the transverse axis, and the point c on the conju-
gate axis, any required number of points may be found.
4th Method.—By the above method, large curves may be
described continuously, by means of a bar, m k, with steel
(Fig. 144.)
points m, l, k, rivetted into brass slides, adjusted to the
length of the semi-axes, and fixed with set-screws. A rect-
angular cross E G, with guiding slots, is placed to coincide
31
off B E equal to the side B C of the rectangle ; bisect A E
at K, and describe a semicircle on A E ; draw the perpen-
dicular B H, cutting the circle at H, and on B H describe
the square B G required.
SECTION Y.
Problems on the Ellipse and the Parabola.
The Ellipse.
Definitions.—An ellipse (Fig. 141) is an oval-shaped
curve. The line A B that divides it into two equal and
symmetrical parts, is the transverse axis. The line D E,
perpendicular to the transverse at its centre point C, is
the conjugate axis. The points A, B, are the vertices of
the curve. The points F, and F', in the transverse axis,
are the foci of the curve, each being termed a focus ; and
they are so placed that their united distances from either
end of the conjugate axis, are equal together to the length
of the transverse axis. By the same rule, the distance of
each focus from the end of the conjugate axis, is equal to
the half-length of the transverse axis.
The ellipse has the characteristic property, that the
sum of the distances of any point in the curve from the
foci, is equal to the transverse axis ; so that the distances,
jointly, of all the points in the curve from the foci, are
equal.
Problem L.—To describe an ellipse, the length and
breadth, or the two axes, being given.
1st Method.—Bisect the transverse axis A B at C, and
through C draw the perpendicular D E, making C D and
C E each equal to half the conjugate diameter. On D as
a centre, with C A as radius, describe arcs cutting at
F, F', for the foci. Divide A C into a number of parts at
the points 1, 2, 3, &c. With radius A 1, on F and F' as
centres, describe arcs; and with radius B 1, on the same
centres, describe arcs intersecting the others as shown.
Repeat the operation for the other divisions of the trans-
verse axis. The series of intersections thus found will
be points in the curve, and they may be as numerously
found as is desirable ; after which a curve traced through
them will form the complete ellipse.
2d Method.—The two axes, A B, D E, being given.
On A B and D E, as diameters, from the same centre C,
describe circles F G, H I; take a convenient number of
points, a, b, &c., in the semi-circumference A F B, and
draw radii cutting the inner circle at a', b', &c.; from
a, b, &c., draw perpendiculars to A B and from a', b\
(.Fig. 140.)
&c., draw parallels to A B, cutting the respective perpen-
diculars at n, o, &c. The points of intersection so found,
are points in the curve.
3d Method.—Along the straight edge of a slip of stiff
paper, mark off a distance a c equal to A C, half the trans-
verse axis; and from the same point a distance a b equal
(Fig. 143.)
to C D, half the conjugate axis. Place the slip so as to
bring the point b on the line A B of the transverse axis,
and the point c on the line D E; and set off on the draw-
ing the position of the point a. Always keeping the point
b on the transverse axis, and the point c on the conju-
gate axis, any required number of points may be found.
4th Method.—By the above method, large curves may be
described continuously, by means of a bar, m k, with steel
(Fig. 144.)
points m, l, k, rivetted into brass slides, adjusted to the
length of the semi-axes, and fixed with set-screws. A rect-
angular cross E G, with guiding slots, is placed to coincide