ENGINEER AND MACHINIST’S DRAWING-BOOK.
QO
with the two axes of the ellipse A C and BH; by sliding
the points h, l, in the slots, and carrying round the point
m, the curve may be completely described. If desirable,
of course, a pen or pencil may be fixed at m.
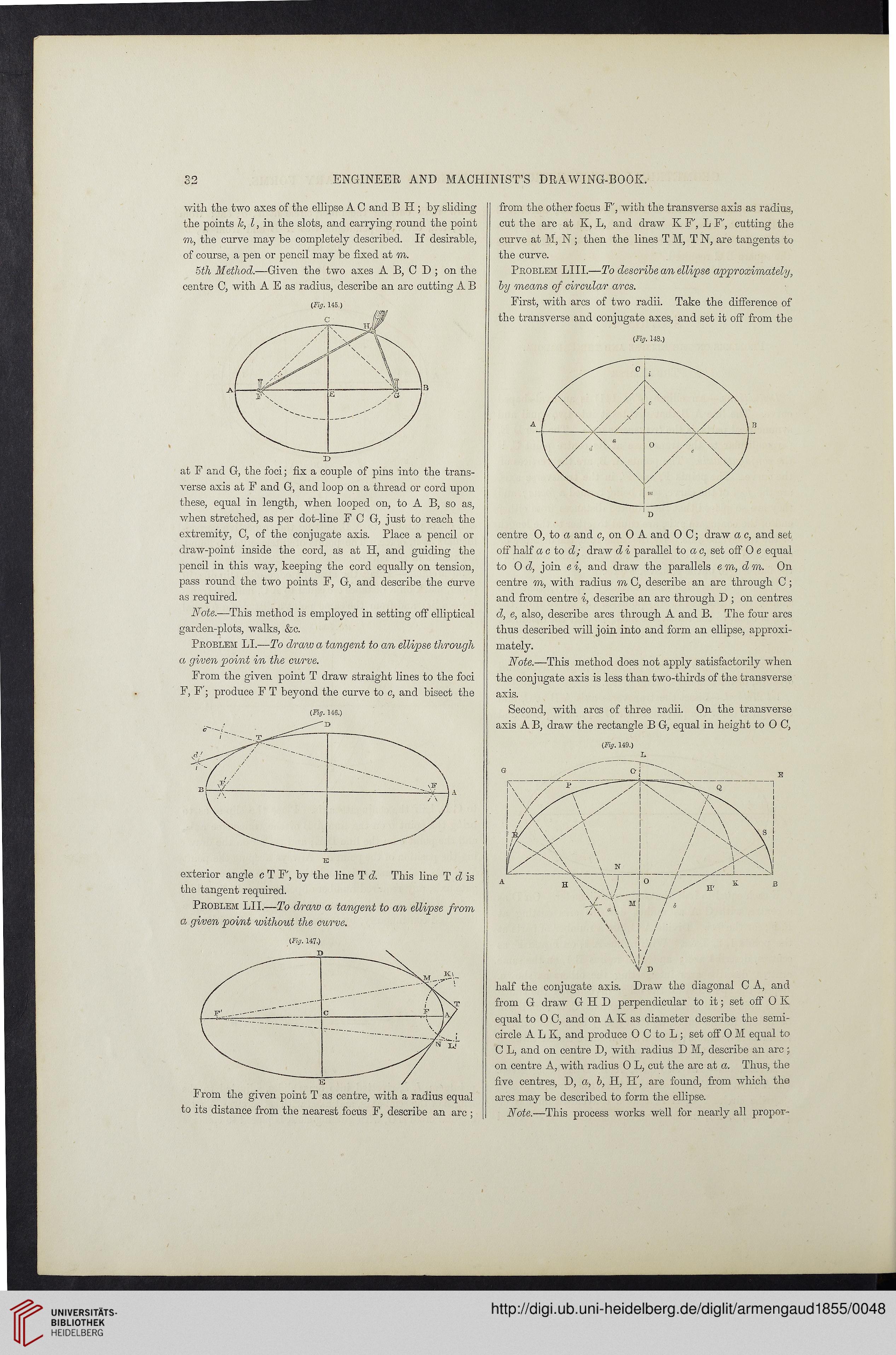
5th Method.—Given the two axes A B, C D ; on the
centre C, with A E as radius, describe an arc cutting A B
(Fig. 145.)
at F and G, the foci; fix a couple of pins into the trans-
verse axis at F and G, and loop on a thread or cord upon
these, equal in length, when looped on, to A B, so as,
when stretched, as per dot-line F C G, just to reach the
extremity, C, of the conjugate axis. Place a pencil or
draw-point inside the cord, as at H, and guiding the
pencil in this way, keeping the cord equally on tension,
pass round the two points F, G, and describe the curve
as required.
Note.—This method is employed in setting off elliptical
garden-plots, walks, &c.
Problem LI.—To draw a tangent to an ellipse through
a given point in the curve.
From the given point T draw straight lines to the foci
F, F; produce F T beyond the curve to c, and bisect the
exterior angle c T F', by the line T d. This line T d is
the tangent required.
Problem LII.—To draw a tangent to an ellipse from
a given point without the curve.
(Fig. 147.)
From the given point T as centre, with a radius equal
to its distance from the nearest focus F, describe an arc ;
from the other focus F', with the transverse axis as radius,
cut the arc at K, L, and draw K F', L F', cutting the
curve at M, N ; then the lines T M, T N, are tangents to
the curve.
Problem LIII.—To describe an ellipse approximately,
by means of circular arcs.
First, with arcs of two radii. Take the difference of
the transverse and conjugate axes, and set it off from the
(Fig. 148.)
centre 0, to a and c, on 0 A and O C; draw a c, and set
off half a c to cl; draw d i parallel to a c, set off 0 e equal
to 0 d, join e i, and draw the parallels em, d m. On
centre to, with radius to C, describe an arc through C;
and from centre i, describe an arc through D ; on centres
cl, e, also, describe arcs through A and B. The four arcs
thus described will join into and form an ellipse, approxi-
mately.
Note.—This method does not apply satisfactorily when
the conjugate axis is less than two-thirds of the transverse
axis.
Second, with arcs of three radii. On the transverse
axis A B, draw the rectangle B G, equal in height to 0 C,
(Fig. 149.)
n
half the conjugate axis. Draw the diagonal 0 A, and
from G draw G H D perpendicular to it; set off 0 K
equal to 0 C, and on A K as diameter describe the semi-
circle A L K, and produce 0 C to L ; set off 0 M equal to
0 L, and on centre D, with radius D M, describe an arc;
on centre A, with radius 0 L, cut the arc at a. Thus, the
five centres, D, a, b, H, PI', are found, from which the
arcs may be described to form the ellipse.
Note.-—This process works well for nearly all proper-
QO
with the two axes of the ellipse A C and BH; by sliding
the points h, l, in the slots, and carrying round the point
m, the curve may be completely described. If desirable,
of course, a pen or pencil may be fixed at m.
5th Method.—Given the two axes A B, C D ; on the
centre C, with A E as radius, describe an arc cutting A B
(Fig. 145.)
at F and G, the foci; fix a couple of pins into the trans-
verse axis at F and G, and loop on a thread or cord upon
these, equal in length, when looped on, to A B, so as,
when stretched, as per dot-line F C G, just to reach the
extremity, C, of the conjugate axis. Place a pencil or
draw-point inside the cord, as at H, and guiding the
pencil in this way, keeping the cord equally on tension,
pass round the two points F, G, and describe the curve
as required.
Note.—This method is employed in setting off elliptical
garden-plots, walks, &c.
Problem LI.—To draw a tangent to an ellipse through
a given point in the curve.
From the given point T draw straight lines to the foci
F, F; produce F T beyond the curve to c, and bisect the
exterior angle c T F', by the line T d. This line T d is
the tangent required.
Problem LII.—To draw a tangent to an ellipse from
a given point without the curve.
(Fig. 147.)
From the given point T as centre, with a radius equal
to its distance from the nearest focus F, describe an arc ;
from the other focus F', with the transverse axis as radius,
cut the arc at K, L, and draw K F', L F', cutting the
curve at M, N ; then the lines T M, T N, are tangents to
the curve.
Problem LIII.—To describe an ellipse approximately,
by means of circular arcs.
First, with arcs of two radii. Take the difference of
the transverse and conjugate axes, and set it off from the
(Fig. 148.)
centre 0, to a and c, on 0 A and O C; draw a c, and set
off half a c to cl; draw d i parallel to a c, set off 0 e equal
to 0 d, join e i, and draw the parallels em, d m. On
centre to, with radius to C, describe an arc through C;
and from centre i, describe an arc through D ; on centres
cl, e, also, describe arcs through A and B. The four arcs
thus described will join into and form an ellipse, approxi-
mately.
Note.—This method does not apply satisfactorily when
the conjugate axis is less than two-thirds of the transverse
axis.
Second, with arcs of three radii. On the transverse
axis A B, draw the rectangle B G, equal in height to 0 C,
(Fig. 149.)
n
half the conjugate axis. Draw the diagonal 0 A, and
from G draw G H D perpendicular to it; set off 0 K
equal to 0 C, and on A K as diameter describe the semi-
circle A L K, and produce 0 C to L ; set off 0 M equal to
0 L, and on centre D, with radius D M, describe an arc;
on centre A, with radius 0 L, cut the arc at a. Thus, the
five centres, D, a, b, H, PI', are found, from which the
arcs may be described to form the ellipse.
Note.-—This process works well for nearly all proper-