96
ENGINEER AND MACHINIST’S DRAWING-BOOK.
PART EIGHTH.
PERSPECTIVE.
SECTION I.
As an introduction to this study, it is necessary to ob-
serve, that a luminous point emits rays in all directions,
and that all the points of the surface of a body are ren-
dered visible by means of rays, which represent the axes
of different cones formed by the emanation of bundles of
rays from these points.
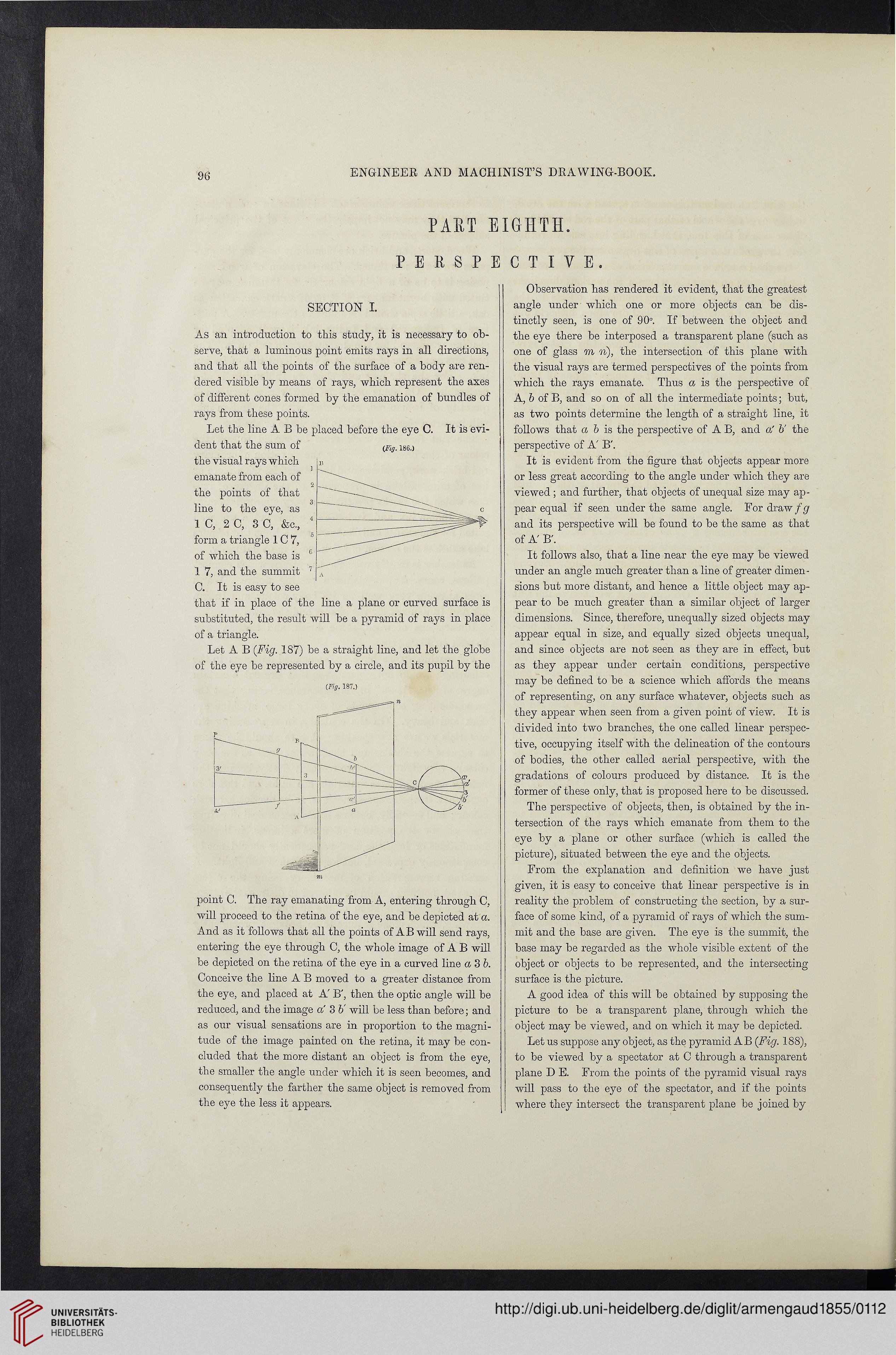
Let the line A B be placed before the eye C. It is evi-
dent that the sum of ^ 186)
the visual rays which
emanate from each of
2
the points of that
3
line to the eye, as
1 C, 2 C, 3 C, &c., 4
form a triangle 1C 7,
of which the base is f'
1 7, and the summit 7
C. It is easy to see
that if in place of the line a plane or curved surface is
substituted, the result will be a pyramid of rays in place
of a triangle.
Let A B (Fig. 187) be a straight line, and let the globe
of the eye be represented by a circle, and its pupil by the
(Fig. 187.)
point C. The ray emanating from A, entering through C,
will proceed to the retina of the eye, and be depicted at a.
And as it follows that all the points of AB will send rays,
entering the eye through C, the whole image of A B will
be depicted on the retina of the eye in a curved line a 3 b.
Conceive the line A B moved to a greater distance from
the eye, and placed at A' B', then the optic angle will be
reduced, and the image ci 3 b' will be less than before; and
as our visual sensations are in proportion to the magni-
tude of the image painted on the retina, it may be con-
cluded that the more distant an object is from the eye,
the smaller the angle under which it is seen becomes, and
consequently the farther the same object is removed from
the eye the less it appears.
Observation has rendered it evident, that the greatest
angle under which one or more objects can be dis-
tinctly seen, is one of 90°. If between the object and
the eye there be interposed a transparent plane (such as
one of glass m n), the intersection of this plane with
the visual rays are termed perspectives of the points from
which the rays emanate. Thus a is the perspective of
A, b of B, and so on of all the intermediate points; but,
as two points determine the length of a straight line, it
follows that a b is the perspective of A B, and a' b' the
perspective of A' B\
It is evident from the figure that objects appear more
or less great according to the angle under which they are
viewed; and further, that objects of unequal size may ap-
pear equal if seen under the same angle. For draw fg
and its perspective will be found to be the same as that
of A' B\
It follows also, that a line near the eye may be viewed
under an angle much greater than a line of greater dimen-
sions but more distant, and hence a little object may ap-
pear to be much greater than a similar object of larger
dimensions. Since, therefore, unequally sized objects may
appear equal in size, and equally sized objects unequal,
and since objects are not seen as they are in effect, but
as they appear under certain conditions, perspective
may be defined to be a science which affords the means
of representing, on any surface whatever, objects such as
they appear when seen from a given point of view. It is
divided into two branches, the one called linear perspec-
tive, occupying itself with the delineation of the contours
of bodies, the other called aerial perspective, with the
gradations of colours produced by distance. It is the
former of these only, that is proposed here to be discussed.
The perspective of objects, then, is obtained by the in-
tersection of the rays which emanate from them to the
eye by a plane or other surface (which is called the
picture), situated between the eye and the objects.
From the explanation and definition we have just
given, it is easy to conceive that linear perspective is in
reality the problem of constructing the section, by a sur-
face of some kind, of a pyramid of rays of which the sum-
mit and the base are given. The eye is the summit, the
base may be regarded as the whole visible extent of the
object or objects to be represented, and the intersecting
surface is the picture.
A good idea of this will be obtained by supposing the
picture to be a transparent plane, through which the
object may be viewed, and on which it may be depicted.
Let us suppose any object, as the pyramid AB (Fig. 188),
to be viewed by a spectator at C through a transparent
plane D E. From the points of the pyramid visual rays
will pass to the eye of the spectator, and if the points
where they intersect the transparent plane be joined by
ENGINEER AND MACHINIST’S DRAWING-BOOK.
PART EIGHTH.
PERSPECTIVE.
SECTION I.
As an introduction to this study, it is necessary to ob-
serve, that a luminous point emits rays in all directions,
and that all the points of the surface of a body are ren-
dered visible by means of rays, which represent the axes
of different cones formed by the emanation of bundles of
rays from these points.
Let the line A B be placed before the eye C. It is evi-
dent that the sum of ^ 186)
the visual rays which
emanate from each of
2
the points of that
3
line to the eye, as
1 C, 2 C, 3 C, &c., 4
form a triangle 1C 7,
of which the base is f'
1 7, and the summit 7
C. It is easy to see
that if in place of the line a plane or curved surface is
substituted, the result will be a pyramid of rays in place
of a triangle.
Let A B (Fig. 187) be a straight line, and let the globe
of the eye be represented by a circle, and its pupil by the
(Fig. 187.)
point C. The ray emanating from A, entering through C,
will proceed to the retina of the eye, and be depicted at a.
And as it follows that all the points of AB will send rays,
entering the eye through C, the whole image of A B will
be depicted on the retina of the eye in a curved line a 3 b.
Conceive the line A B moved to a greater distance from
the eye, and placed at A' B', then the optic angle will be
reduced, and the image ci 3 b' will be less than before; and
as our visual sensations are in proportion to the magni-
tude of the image painted on the retina, it may be con-
cluded that the more distant an object is from the eye,
the smaller the angle under which it is seen becomes, and
consequently the farther the same object is removed from
the eye the less it appears.
Observation has rendered it evident, that the greatest
angle under which one or more objects can be dis-
tinctly seen, is one of 90°. If between the object and
the eye there be interposed a transparent plane (such as
one of glass m n), the intersection of this plane with
the visual rays are termed perspectives of the points from
which the rays emanate. Thus a is the perspective of
A, b of B, and so on of all the intermediate points; but,
as two points determine the length of a straight line, it
follows that a b is the perspective of A B, and a' b' the
perspective of A' B\
It is evident from the figure that objects appear more
or less great according to the angle under which they are
viewed; and further, that objects of unequal size may ap-
pear equal if seen under the same angle. For draw fg
and its perspective will be found to be the same as that
of A' B\
It follows also, that a line near the eye may be viewed
under an angle much greater than a line of greater dimen-
sions but more distant, and hence a little object may ap-
pear to be much greater than a similar object of larger
dimensions. Since, therefore, unequally sized objects may
appear equal in size, and equally sized objects unequal,
and since objects are not seen as they are in effect, but
as they appear under certain conditions, perspective
may be defined to be a science which affords the means
of representing, on any surface whatever, objects such as
they appear when seen from a given point of view. It is
divided into two branches, the one called linear perspec-
tive, occupying itself with the delineation of the contours
of bodies, the other called aerial perspective, with the
gradations of colours produced by distance. It is the
former of these only, that is proposed here to be discussed.
The perspective of objects, then, is obtained by the in-
tersection of the rays which emanate from them to the
eye by a plane or other surface (which is called the
picture), situated between the eye and the objects.
From the explanation and definition we have just
given, it is easy to conceive that linear perspective is in
reality the problem of constructing the section, by a sur-
face of some kind, of a pyramid of rays of which the sum-
mit and the base are given. The eye is the summit, the
base may be regarded as the whole visible extent of the
object or objects to be represented, and the intersecting
surface is the picture.
A good idea of this will be obtained by supposing the
picture to be a transparent plane, through which the
object may be viewed, and on which it may be depicted.
Let us suppose any object, as the pyramid AB (Fig. 188),
to be viewed by a spectator at C through a transparent
plane D E. From the points of the pyramid visual rays
will pass to the eye of the spectator, and if the points
where they intersect the transparent plane be joined by