98
ENGINEER AND MACHINIST’S DRAWING-BOOK
right angles in the line H I, is called the horizontal plane,
and its intersection with the plane of the picture is called
the horizon line, the horizon of the picture, or simply the
horizon.
4. The plane M N passing vertically through the eye of
the spectator, and cutting each of the other planes in a
right angle, is called the vertical plane, and sometimes the
central plane; hut as the term vertical plane is applied
to any plane that is perpendicular to the ground plane,
we shall use the term central plane for the sake of avoid-
ing confusion; for the same reason, and before proceeding
further, it is necessary to premise a few definitions.
Point of view, or point of sight, is the point where the
eye is supposed to be placed to view the object, as at 0
(Pig. 189), and is the vertex of the optic cone. Its pro-
jection on the ground plane in Fig. 190 is M, and is termed
the station point.
The projection of any point on the ground plane is
called the seat of that point.
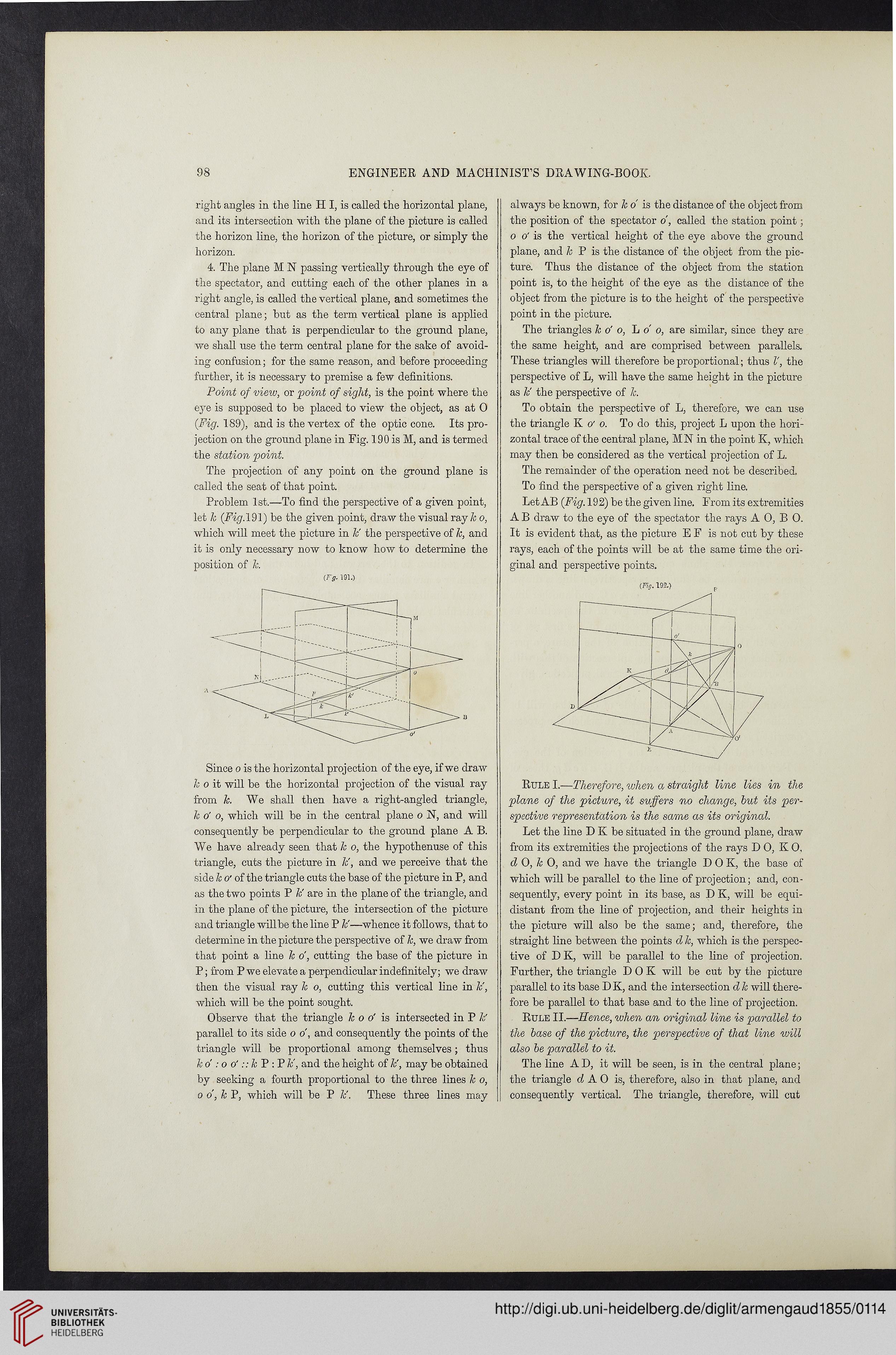
Problem 1st.—To find the perspective of a given point,
let h (Fig. 191) be the given point, draw the visual ray k o,
which will meet the picture in 1c the perspective of k, and
it is only necessary now to know how to determine the
position of k.
(Fff. 191.)
Since o is the horizontal projection of the eye, if we draw
k o it will be the horizontal projection of the visual ray
from k. We shall then have a right-angled triangle,
k o' o, which will be in the central plane o N, and will
consequently be perpendicular to the ground plane A B.
We have already seen that k o, the hypothenuse of this
triangle, cuts the picture in k', and we perceive that the
side k o’ of the triangle cuts the base of the picture in P, and
as the two points P k' are in the plane of the triangle, and
in the plane of the picture, the intersection of the picture
and triangle will be the line P k’—whence it follows, that to
determine in the picture the perspective of k, we draw from
that point a line k o', cutting the base of the picture in
P; from P we elevate a perpendicular indefinitely; we draw
then the visual ray k o, cutting this vertical line in k',
which will be the point sought.
Observe that the triangle k o o' is intersected in P k!
parallel to its side o o, and consequently the points of the
triangle will be proportional among themselves ; thus
k o': o o':: k P : P k', and the height of k', may be obtained
by seeking a fourth proportional to the three lines k o,
o o', k P, which will be P k'. These three lines may
always be known, for k 6 is the distance of the object from
the position of the spectator o', called the station point;
o o' is the vertical height of the eye above the ground
plane, and k P is the distance of the object from the pic-
ture. Thus the distance of the object from the station
point is, to the height of the eye as the distance of the
object from the picture is to the height of the perspective
point in the picture.
The triangles k o' o, L o' o, are similar, since they are
the same height, and are comprised between parallels.
These triangles will therefore be proportional; thus V, the
perspective of L, will have the same height in the picture
as k‘ the perspective of k.
To obtain the perspective of L, therefore, we can use
the triangle K o’ o. To do this, project L upon the hori-
zontal trace of the central plane, MN in the point K, which
may then be considered as the vertical projection of L.
The remainder of the operation need not be described.
To find the perspective of a given right line.
Let AB (Fig. 192) be the given line. From its extremities
A B draw to the eye of the spectator the rays A 0, B O.
It is evident that, as the picture E F is not cut by these
rays, each of the points will be at the same time the ori-
ginal and perspective points.
(Fig. 192.)
Rule I.—Therefore, when a straight line lies in the
plane of the picture, it suffers no change, but its per-
spective representation is the same as its original.
Let the line D K be situated in the ground plane, draw
from its extremities the projections of the rays D 0, K 0,
d O, k O, and we have the triangle D O K, the base of
which will be parallel to the line of projection; and, con-
sequently, every point in its base, as D K, will be equi-
distant from the line of projection, and their heights in
the picture will also be the same; and, therefore, the
straight line between the points d k, which is the perspec-
tive of D K, will be parallel to the line of projection.
Further, the triangle D 0 K will be cut by the picture
parallel to its base D K, and the intersection d k will there-
fore be parallel to that base and to the line of projection.
Rule II.—Hence, when an original line is parallel to
the base of the picture, the perspective of that line will
also be parallel to it.
The line A D, it will be seen, is in the central plane;
the triangle d A O is, therefore, also in that plane, and
consequently vertical. The triangle, therefore, will cut
ENGINEER AND MACHINIST’S DRAWING-BOOK
right angles in the line H I, is called the horizontal plane,
and its intersection with the plane of the picture is called
the horizon line, the horizon of the picture, or simply the
horizon.
4. The plane M N passing vertically through the eye of
the spectator, and cutting each of the other planes in a
right angle, is called the vertical plane, and sometimes the
central plane; hut as the term vertical plane is applied
to any plane that is perpendicular to the ground plane,
we shall use the term central plane for the sake of avoid-
ing confusion; for the same reason, and before proceeding
further, it is necessary to premise a few definitions.
Point of view, or point of sight, is the point where the
eye is supposed to be placed to view the object, as at 0
(Pig. 189), and is the vertex of the optic cone. Its pro-
jection on the ground plane in Fig. 190 is M, and is termed
the station point.
The projection of any point on the ground plane is
called the seat of that point.
Problem 1st.—To find the perspective of a given point,
let h (Fig. 191) be the given point, draw the visual ray k o,
which will meet the picture in 1c the perspective of k, and
it is only necessary now to know how to determine the
position of k.
(Fff. 191.)
Since o is the horizontal projection of the eye, if we draw
k o it will be the horizontal projection of the visual ray
from k. We shall then have a right-angled triangle,
k o' o, which will be in the central plane o N, and will
consequently be perpendicular to the ground plane A B.
We have already seen that k o, the hypothenuse of this
triangle, cuts the picture in k', and we perceive that the
side k o’ of the triangle cuts the base of the picture in P, and
as the two points P k' are in the plane of the triangle, and
in the plane of the picture, the intersection of the picture
and triangle will be the line P k’—whence it follows, that to
determine in the picture the perspective of k, we draw from
that point a line k o', cutting the base of the picture in
P; from P we elevate a perpendicular indefinitely; we draw
then the visual ray k o, cutting this vertical line in k',
which will be the point sought.
Observe that the triangle k o o' is intersected in P k!
parallel to its side o o, and consequently the points of the
triangle will be proportional among themselves ; thus
k o': o o':: k P : P k', and the height of k', may be obtained
by seeking a fourth proportional to the three lines k o,
o o', k P, which will be P k'. These three lines may
always be known, for k 6 is the distance of the object from
the position of the spectator o', called the station point;
o o' is the vertical height of the eye above the ground
plane, and k P is the distance of the object from the pic-
ture. Thus the distance of the object from the station
point is, to the height of the eye as the distance of the
object from the picture is to the height of the perspective
point in the picture.
The triangles k o' o, L o' o, are similar, since they are
the same height, and are comprised between parallels.
These triangles will therefore be proportional; thus V, the
perspective of L, will have the same height in the picture
as k‘ the perspective of k.
To obtain the perspective of L, therefore, we can use
the triangle K o’ o. To do this, project L upon the hori-
zontal trace of the central plane, MN in the point K, which
may then be considered as the vertical projection of L.
The remainder of the operation need not be described.
To find the perspective of a given right line.
Let AB (Fig. 192) be the given line. From its extremities
A B draw to the eye of the spectator the rays A 0, B O.
It is evident that, as the picture E F is not cut by these
rays, each of the points will be at the same time the ori-
ginal and perspective points.
(Fig. 192.)
Rule I.—Therefore, when a straight line lies in the
plane of the picture, it suffers no change, but its per-
spective representation is the same as its original.
Let the line D K be situated in the ground plane, draw
from its extremities the projections of the rays D 0, K 0,
d O, k O, and we have the triangle D O K, the base of
which will be parallel to the line of projection; and, con-
sequently, every point in its base, as D K, will be equi-
distant from the line of projection, and their heights in
the picture will also be the same; and, therefore, the
straight line between the points d k, which is the perspec-
tive of D K, will be parallel to the line of projection.
Further, the triangle D 0 K will be cut by the picture
parallel to its base D K, and the intersection d k will there-
fore be parallel to that base and to the line of projection.
Rule II.—Hence, when an original line is parallel to
the base of the picture, the perspective of that line will
also be parallel to it.
The line A D, it will be seen, is in the central plane;
the triangle d A O is, therefore, also in that plane, and
consequently vertical. The triangle, therefore, will cut