\
PERSPECTIVE.
103
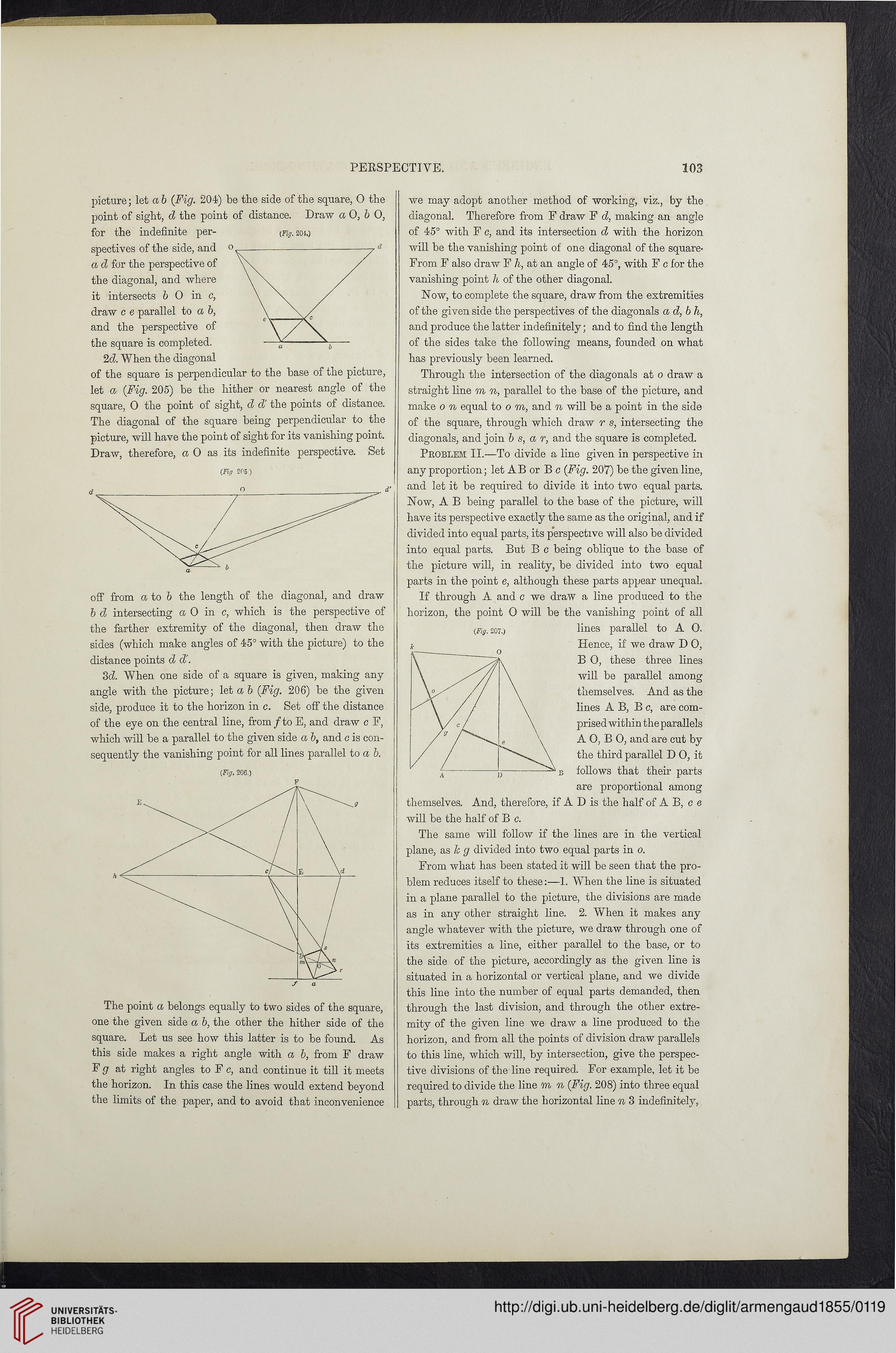
picture; let ab (Fig. 204) be the side of the square, O the
point of sight, d the point of distance. Draw a 0, b O,
for the indefinite per-
spectives of the side, and
a d for the perspective of
the diagonal, and where
it intersects 6 0 in c,
draw c e parallel to a 6,
and the perspective of
the square is completed.
2d. When the diagonal
of the square is perpendicular to the base of the picture,
let a (Fig. 205) be the hither or nearest angle of the
square, 0 the point of sight, d d' the points of distance.
The diagonal of the square being perpendicular to the
picture, will have the point of sight for its vanishing point.
Draw, therefore, a 0 as its indefinite perspective. Set
(Fig 205 )
off from a to 6 the length of the diagonal, and draw
6 d intersecting a 0 in c, which is the perspective of
the farther extremity of the diagonal, then draw the
sides (which make angles of 45° with the picture) to the
distance points d d'.
2d. When one side of a square is given, making any
angle with the picture; let a b (Fig. 206) be the given
side, produce it to the horizon in c. Set off the distance
of the eye on the central line, from / to E, and draw c F,
which will be a parallel to the given side a 6, and c is con-
sequently the vanishing point for all lines parallel to a b.
(Fig. 206.)
The point a belongs equally to two sides of the square,
one the given side a 6, the other the hither side of the
square. Let us see how this latter is to be found. As
this side makes a right angle with a b, from F draw
F gr at right angles to F c, and continue it till it meets
the horizon. In this case the lines would extend beyond
the limits of the paper, and to avoid that inconvenience
(Fig. 207.)
we may adopt another method of working, viz., by the
diagonal. Therefore from F draw F d, making an angle
of 45° with F c, and its intersection d with the horizon
will be the vanishing point of one diagonal of the square*
From F also draw F h, at an angle of 45°, with F c for the
vanishing point h of the other diagonal.
Now, to complete the square, draw from the extremities
of the given side the perspectives of the diagonals a d, b h,
and produce the latter indefinitely; and to find the length
of the sides take the following means, founded on what
has previously been learned.
Through the intersection of the diagonals at o draw a
straight line m n, parallel to the base of the picture, and
make o n equal to o m, and n will be a point in the side
of the square, through which draw r s, intersecting the
diagonals, and join 6 s, a r, and the square is completed.
Problem II.—To divide a line given in perspective in
any proportion; let A B or Be (Fig. 207) be the given line,
and let it be required to divide it into two equal parts.
Now, A B being parallel to the base of the picture, will
have its perspective exactly the same as the original, and if
divided into equal parts, its perspective will also be divided
into equal parts. But B c being oblique to the base of
the picture will, in reality, be divided into two equal
parts in the point e, although these parts appear unequal.
If through A and c we draw a line produced to the
horizon, the point O will be the vanishing point of all
lines parallel to A O.
Hence, if' we draw D O,
B O, these three lines
will be parallel among
themselves. And as the
lines A B, Be, are com-
prised within the parallels
AO, BO, and are cut by
the third parallel D O, it
follows that their parts
are proportional among
themselves. And, therefore, if A D is the half of A B, c e
will be the half of B c.
The same will follow if the lines are in the vertical
plane, as kg divided into two equal parts in o.
From what has been stated it will be seen that the pro-
blem reduces itself to these:—1. When the line is situated
in a plane parallel to the picture, the divisions are made
as in any other straight line. 2. When it makes any
angle whatever with the picture, we draw through one of
its extremities a line, either parallel to the base, or to
the side of the picture, accordingly as the given line is
situated in a horizontal or vertical plane, and we divide
this line into the number of equal parts demanded, then
through the last division, and through the other extre-
mity of the given line we draw a line produced to the
horizon, and from all the points of division draw parallels
to this line, which will, by intersection, give the perspec-
tive divisions of the line required. For example, let it be
required to divide the line m n (Fig. 208) into three equal
parts, through n draw the horizontal line n 3 indefinitely,
PERSPECTIVE.
103
picture; let ab (Fig. 204) be the side of the square, O the
point of sight, d the point of distance. Draw a 0, b O,
for the indefinite per-
spectives of the side, and
a d for the perspective of
the diagonal, and where
it intersects 6 0 in c,
draw c e parallel to a 6,
and the perspective of
the square is completed.
2d. When the diagonal
of the square is perpendicular to the base of the picture,
let a (Fig. 205) be the hither or nearest angle of the
square, 0 the point of sight, d d' the points of distance.
The diagonal of the square being perpendicular to the
picture, will have the point of sight for its vanishing point.
Draw, therefore, a 0 as its indefinite perspective. Set
(Fig 205 )
off from a to 6 the length of the diagonal, and draw
6 d intersecting a 0 in c, which is the perspective of
the farther extremity of the diagonal, then draw the
sides (which make angles of 45° with the picture) to the
distance points d d'.
2d. When one side of a square is given, making any
angle with the picture; let a b (Fig. 206) be the given
side, produce it to the horizon in c. Set off the distance
of the eye on the central line, from / to E, and draw c F,
which will be a parallel to the given side a 6, and c is con-
sequently the vanishing point for all lines parallel to a b.
(Fig. 206.)
The point a belongs equally to two sides of the square,
one the given side a 6, the other the hither side of the
square. Let us see how this latter is to be found. As
this side makes a right angle with a b, from F draw
F gr at right angles to F c, and continue it till it meets
the horizon. In this case the lines would extend beyond
the limits of the paper, and to avoid that inconvenience
(Fig. 207.)
we may adopt another method of working, viz., by the
diagonal. Therefore from F draw F d, making an angle
of 45° with F c, and its intersection d with the horizon
will be the vanishing point of one diagonal of the square*
From F also draw F h, at an angle of 45°, with F c for the
vanishing point h of the other diagonal.
Now, to complete the square, draw from the extremities
of the given side the perspectives of the diagonals a d, b h,
and produce the latter indefinitely; and to find the length
of the sides take the following means, founded on what
has previously been learned.
Through the intersection of the diagonals at o draw a
straight line m n, parallel to the base of the picture, and
make o n equal to o m, and n will be a point in the side
of the square, through which draw r s, intersecting the
diagonals, and join 6 s, a r, and the square is completed.
Problem II.—To divide a line given in perspective in
any proportion; let A B or Be (Fig. 207) be the given line,
and let it be required to divide it into two equal parts.
Now, A B being parallel to the base of the picture, will
have its perspective exactly the same as the original, and if
divided into equal parts, its perspective will also be divided
into equal parts. But B c being oblique to the base of
the picture will, in reality, be divided into two equal
parts in the point e, although these parts appear unequal.
If through A and c we draw a line produced to the
horizon, the point O will be the vanishing point of all
lines parallel to A O.
Hence, if' we draw D O,
B O, these three lines
will be parallel among
themselves. And as the
lines A B, Be, are com-
prised within the parallels
AO, BO, and are cut by
the third parallel D O, it
follows that their parts
are proportional among
themselves. And, therefore, if A D is the half of A B, c e
will be the half of B c.
The same will follow if the lines are in the vertical
plane, as kg divided into two equal parts in o.
From what has been stated it will be seen that the pro-
blem reduces itself to these:—1. When the line is situated
in a plane parallel to the picture, the divisions are made
as in any other straight line. 2. When it makes any
angle whatever with the picture, we draw through one of
its extremities a line, either parallel to the base, or to
the side of the picture, accordingly as the given line is
situated in a horizontal or vertical plane, and we divide
this line into the number of equal parts demanded, then
through the last division, and through the other extre-
mity of the given line we draw a line produced to the
horizon, and from all the points of division draw parallels
to this line, which will, by intersection, give the perspec-
tive divisions of the line required. For example, let it be
required to divide the line m n (Fig. 208) into three equal
parts, through n draw the horizontal line n 3 indefinitely,