104
ENGINEER AND MACHINIST’S DRAWING-BOOK.
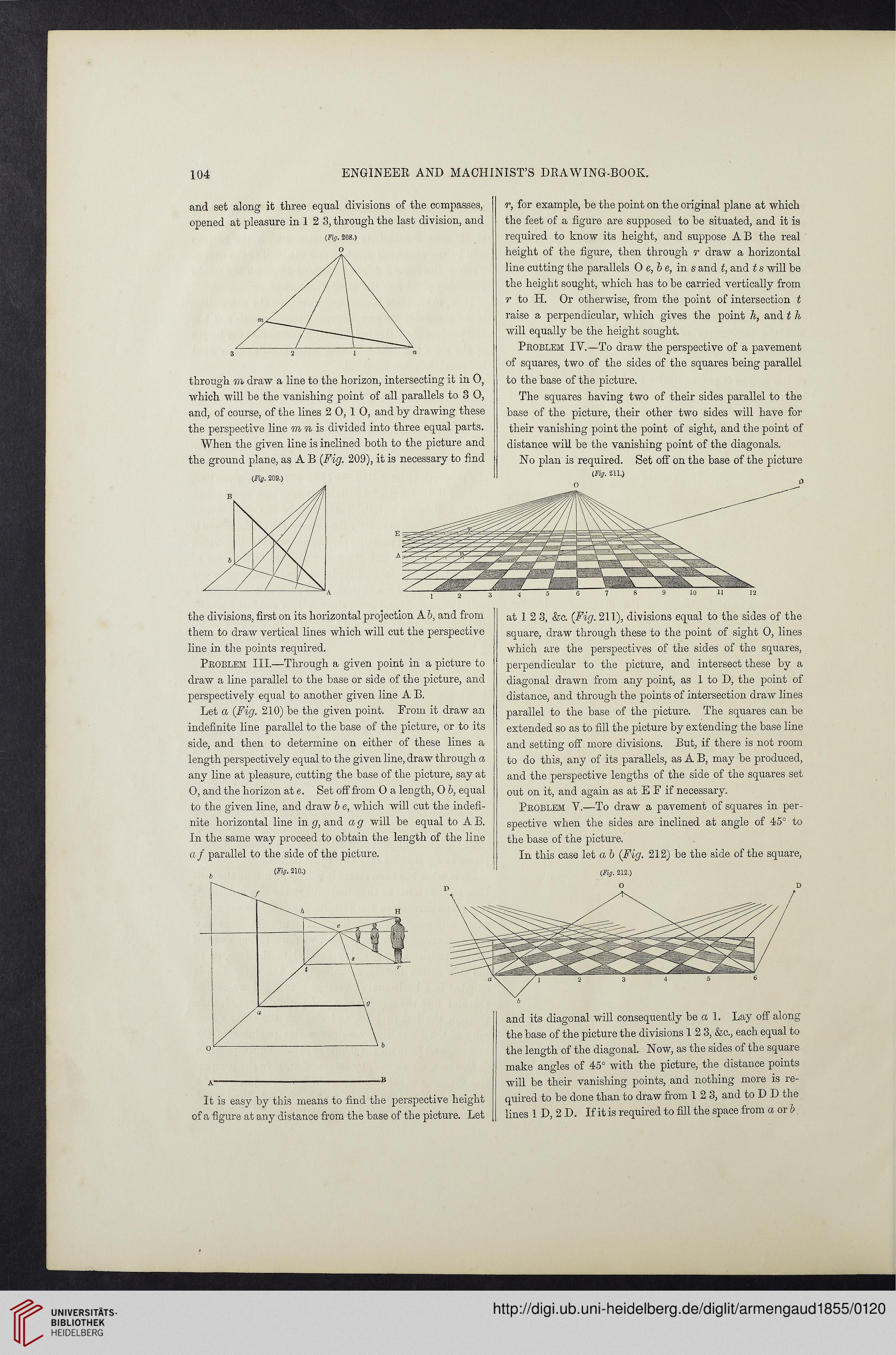
and set along it three equal divisions of the compasses,
opened at pleasure in 1 2 3, through the last division, and
(Fig. 208.)
O
through m draw a line to the horizon, intersecting it in 0,
which will be the vanishing point of all parallels to 3 O,
and, of course, of the lines 2 0, 1 O, and by drawing these
the perspective line m n is divided into three equal parts.
When the given line is inclined both to the picture and
the ground plane, as A B (Fig. 209), it is necessary to find
r, for example, be the point on the original plane at which
the feet of a figure are supposed to be situated, and it is
required to know its height, and suppose AB the real
height of the figure, then through r draw a horizontal
line cutting the parallels O e, b e, in s and t, and t s will be
the height sought, which has to be carried vertically from
r to H. Or otherwise, from the point of intersection t
raise a perpendicular, which gives the point h, and t h
will equally be the height sought.
Problem: IY.—To draw the perspective of a pavement
of squares, two of the sides of the squares being parallel
to the base of the picture.
The squares having two of their sides parallel to the
base of the picture, their other two sides will have for
their vanishing point the point of sight, and the point of
distance will be the vanishing point of the diagonals.
No plan is required. Set off on the base of the picture
(Fig. 209.)
(Fig. 211.)
the divisions, first on its horizontal projection Ah, and from
them to draw vertical lines which will cut the perspective
line in the points required.
Problem III.—Through a given point in a picture to
draw a line parallel to the base or side of the picture, and
perspectively equal to another given line A B.
Let a (Fig. 210) be the given point. From it draw an
indefinite line parallel to the base of the picture, or to its
side, and then to determine on either of these lines a
length perspectively equal to the given line, draw through a
any line at pleasure, cutting the base of the picture, say at
O, and the horizon at e. Set off from O a length, O b, equal
to the given line, and draw b e, which will cut the indefi-
nite horizontal line in g, and a g will be equal to A B.
In the same way proceed to obtain the length of the line
a f parallel to the side of the picture.
. (Fig. 210.)
at 1 2 3, &c. (Fig. 211), divisions equal to the sides of the
square, draw through these to the point of sight O, lines
which are the perspectives of the sides of the squares,
perpendicular to the picture, and intersect these by a
diagonal drawn from any point, as 1 to D, the point of
distance, and through the points of intersection draw lines
parallel to the base of the picture. The squares can be
extended so as to fill the picture by extending the base line
and setting off more divisions. But, if there is not room
to do this, any of its parallels, as A B, may be produced,
and the perspective lengths of the side of the squares set
out on it, and again as at E F if necessary.
Problem Y.—To draw a pavement of squares in per-
spective when the sides are inclined at angle of 45° to
the base of the picture.
In this case let a b (Fig. 212) be the side of the square,
(Fig. 212.)
It is easy by this means to find the perspective height
of a figure at any distance from the base of the picture. Let
and its diagonal will consequently be a 1. Lay off along
the base of the picture the divisions 12 3, &c., each equal to
the length of the diagonal. Now, as the sides of the square
make angles of 45° with the picture, the distance points
will be their vanishing points, and nothing more is re-
quired to be done than to draw from 12 3, and to D D the
lines 1 D, 2 D. If it is required to fill the space from a or b
ENGINEER AND MACHINIST’S DRAWING-BOOK.
and set along it three equal divisions of the compasses,
opened at pleasure in 1 2 3, through the last division, and
(Fig. 208.)
O
through m draw a line to the horizon, intersecting it in 0,
which will be the vanishing point of all parallels to 3 O,
and, of course, of the lines 2 0, 1 O, and by drawing these
the perspective line m n is divided into three equal parts.
When the given line is inclined both to the picture and
the ground plane, as A B (Fig. 209), it is necessary to find
r, for example, be the point on the original plane at which
the feet of a figure are supposed to be situated, and it is
required to know its height, and suppose AB the real
height of the figure, then through r draw a horizontal
line cutting the parallels O e, b e, in s and t, and t s will be
the height sought, which has to be carried vertically from
r to H. Or otherwise, from the point of intersection t
raise a perpendicular, which gives the point h, and t h
will equally be the height sought.
Problem: IY.—To draw the perspective of a pavement
of squares, two of the sides of the squares being parallel
to the base of the picture.
The squares having two of their sides parallel to the
base of the picture, their other two sides will have for
their vanishing point the point of sight, and the point of
distance will be the vanishing point of the diagonals.
No plan is required. Set off on the base of the picture
(Fig. 209.)
(Fig. 211.)
the divisions, first on its horizontal projection Ah, and from
them to draw vertical lines which will cut the perspective
line in the points required.
Problem III.—Through a given point in a picture to
draw a line parallel to the base or side of the picture, and
perspectively equal to another given line A B.
Let a (Fig. 210) be the given point. From it draw an
indefinite line parallel to the base of the picture, or to its
side, and then to determine on either of these lines a
length perspectively equal to the given line, draw through a
any line at pleasure, cutting the base of the picture, say at
O, and the horizon at e. Set off from O a length, O b, equal
to the given line, and draw b e, which will cut the indefi-
nite horizontal line in g, and a g will be equal to A B.
In the same way proceed to obtain the length of the line
a f parallel to the side of the picture.
. (Fig. 210.)
at 1 2 3, &c. (Fig. 211), divisions equal to the sides of the
square, draw through these to the point of sight O, lines
which are the perspectives of the sides of the squares,
perpendicular to the picture, and intersect these by a
diagonal drawn from any point, as 1 to D, the point of
distance, and through the points of intersection draw lines
parallel to the base of the picture. The squares can be
extended so as to fill the picture by extending the base line
and setting off more divisions. But, if there is not room
to do this, any of its parallels, as A B, may be produced,
and the perspective lengths of the side of the squares set
out on it, and again as at E F if necessary.
Problem Y.—To draw a pavement of squares in per-
spective when the sides are inclined at angle of 45° to
the base of the picture.
In this case let a b (Fig. 212) be the side of the square,
(Fig. 212.)
It is easy by this means to find the perspective height
of a figure at any distance from the base of the picture. Let
and its diagonal will consequently be a 1. Lay off along
the base of the picture the divisions 12 3, &c., each equal to
the length of the diagonal. Now, as the sides of the square
make angles of 45° with the picture, the distance points
will be their vanishing points, and nothing more is re-
quired to be done than to draw from 12 3, and to D D the
lines 1 D, 2 D. If it is required to fill the space from a or b