PERSPECTIVE.
105
draw a 0. 6 0, which will he the perspective direction of
the diagonals of the square, and their intersections with
the perspectives of the-sides produced will give their points.
Problem VI.—To draw the perspective of a pavement of
squares, the sides of which form any angle with the picture.
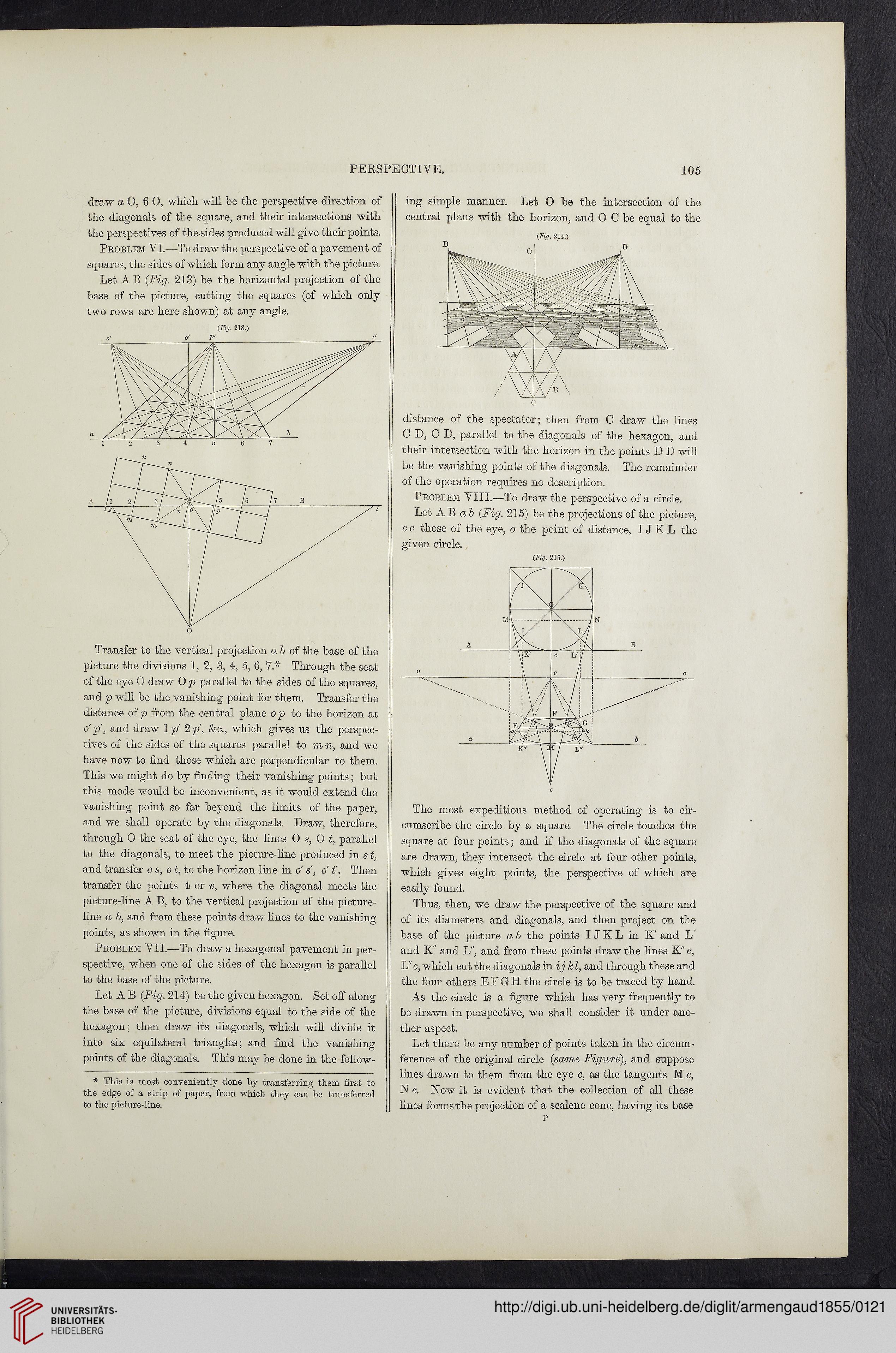
Let AB {Fig. 218) be the horizontal projection of the
base of the picture, cutting the squares (of which only
two rows are here shown) at any angle.
{Fig. 213.)
Transfer to the vertical projection a 1 of the base of the
picture the divisions 1, 2, 3, 4, 5, 6, 7.* Through the seat
of the eye 0 draw Op parallel to the sides of the squares,
and p will be the vanishing point for them. Transfer the
distance of p from the central plane op to the horizon at
o' p, and draw Ip’ 2pi, &c., which gives us the perspec-
tives of the sides of the squares parallel to m n, and we
have now to find those which are perpendicular to them.
This we might do by finding their vanishing points; but
this mode would be inconvenient, as it would extend the
vauishing point so far beyond the limits of the paper,
and we shall operate by the diagonals. Draw, therefore,
through 0 the seat of the eye, the lines 0 s, 0 t, parallel
to the diagonals, to meet the picture-line produced in s t,
and transfer os, ot, to the horizon-line in o' s', o' t'. Then
transfer the points 4 or v, where the diagonal meets the
picture-line A B, to the vertical projection of the picture-
line a b, and from these points draw fines to the vanishing
points, as shown in the figure.
Problem VII.—To draw a hexagonal pavement in per-
spective, when one of the sides of the hexagon is parallel
to the base of the picture.
Let A B {Fig. 214) be the given hexagon. Set off along
the base of the picture, divisions equal to the side of the
hexagon; then draw its diagonals, which will divide it
into six equilateral triangles; and find the vanishing
points of the diagonals. This may be done in the follow-
* This is most conveniently done by transferring them first to
the edge of a strip of paper, from which they can be transferred
to the picture-line.
ing simple manner. Let O be the intersection of the
central plane with the horizon, and 0 C be equal to the
{Fig. 214.)
distance of the spectator; then from C draw the fines
C D, C D, parallel to the diagonals of the hexagon, and
their intersection with the horizon in the points D D will
be the vanishing points of the diagonals. The remainder
of the operation requires no description.
Problem VIII.—To draw the perspective of a circle.
Let A B a b {Fig. 215) be the projections of the picture,
c c those of the eye, o the point of distance, I J K L the
given circle.
(Fig. 215.)
The most expeditious method of operating is to cir-
cumscribe the circle by a square. The circle touches the
square at four points; and if the diagonals of the square
are drawn, they intersect the circle at four other points,
which gives eight points, the perspective of which are
easily found.
Thus, then, we draw the perspective of the square and
of its diameters and diagonals, and then project on the
base of the picture ab the points IJKL in K'and L
and K" and L", and from these points draw the fines K" c,
L"c, which cut the diagonals in ij hi, and through these and
the four others EFGH the circle is to be traced by hand.
As the circle is a figure which has very frequently to
be drawn in perspective, we shall consider it under ano-
ther aspect.
Let there be any number of points taken in the circum-
ference of the original circle {same Figure), and suppose
fines drawn to them from the eye c, as the tangents M c,
N c. Now it is evident that the collection of all these
fines forms the projection of a scalene cone, having its base
p
105
draw a 0. 6 0, which will he the perspective direction of
the diagonals of the square, and their intersections with
the perspectives of the-sides produced will give their points.
Problem VI.—To draw the perspective of a pavement of
squares, the sides of which form any angle with the picture.
Let AB {Fig. 218) be the horizontal projection of the
base of the picture, cutting the squares (of which only
two rows are here shown) at any angle.
{Fig. 213.)
Transfer to the vertical projection a 1 of the base of the
picture the divisions 1, 2, 3, 4, 5, 6, 7.* Through the seat
of the eye 0 draw Op parallel to the sides of the squares,
and p will be the vanishing point for them. Transfer the
distance of p from the central plane op to the horizon at
o' p, and draw Ip’ 2pi, &c., which gives us the perspec-
tives of the sides of the squares parallel to m n, and we
have now to find those which are perpendicular to them.
This we might do by finding their vanishing points; but
this mode would be inconvenient, as it would extend the
vauishing point so far beyond the limits of the paper,
and we shall operate by the diagonals. Draw, therefore,
through 0 the seat of the eye, the lines 0 s, 0 t, parallel
to the diagonals, to meet the picture-line produced in s t,
and transfer os, ot, to the horizon-line in o' s', o' t'. Then
transfer the points 4 or v, where the diagonal meets the
picture-line A B, to the vertical projection of the picture-
line a b, and from these points draw fines to the vanishing
points, as shown in the figure.
Problem VII.—To draw a hexagonal pavement in per-
spective, when one of the sides of the hexagon is parallel
to the base of the picture.
Let A B {Fig. 214) be the given hexagon. Set off along
the base of the picture, divisions equal to the side of the
hexagon; then draw its diagonals, which will divide it
into six equilateral triangles; and find the vanishing
points of the diagonals. This may be done in the follow-
* This is most conveniently done by transferring them first to
the edge of a strip of paper, from which they can be transferred
to the picture-line.
ing simple manner. Let O be the intersection of the
central plane with the horizon, and 0 C be equal to the
{Fig. 214.)
distance of the spectator; then from C draw the fines
C D, C D, parallel to the diagonals of the hexagon, and
their intersection with the horizon in the points D D will
be the vanishing points of the diagonals. The remainder
of the operation requires no description.
Problem VIII.—To draw the perspective of a circle.
Let A B a b {Fig. 215) be the projections of the picture,
c c those of the eye, o the point of distance, I J K L the
given circle.
(Fig. 215.)
The most expeditious method of operating is to cir-
cumscribe the circle by a square. The circle touches the
square at four points; and if the diagonals of the square
are drawn, they intersect the circle at four other points,
which gives eight points, the perspective of which are
easily found.
Thus, then, we draw the perspective of the square and
of its diameters and diagonals, and then project on the
base of the picture ab the points IJKL in K'and L
and K" and L", and from these points draw the fines K" c,
L"c, which cut the diagonals in ij hi, and through these and
the four others EFGH the circle is to be traced by hand.
As the circle is a figure which has very frequently to
be drawn in perspective, we shall consider it under ano-
ther aspect.
Let there be any number of points taken in the circum-
ference of the original circle {same Figure), and suppose
fines drawn to them from the eye c, as the tangents M c,
N c. Now it is evident that the collection of all these
fines forms the projection of a scalene cone, having its base
p