108
ENGINEER AND MACHINIST’S DRAWING-BOOK.
we view an object under an angle of 90°, and we may
consider the sum of the rays which can enter the eye
under that angle as forming a cone, having the eye at
its summit. Now, according to our proposition, the only
objects which can be distinctly seen are those contained
within the base of the cone. A picture is in this con-
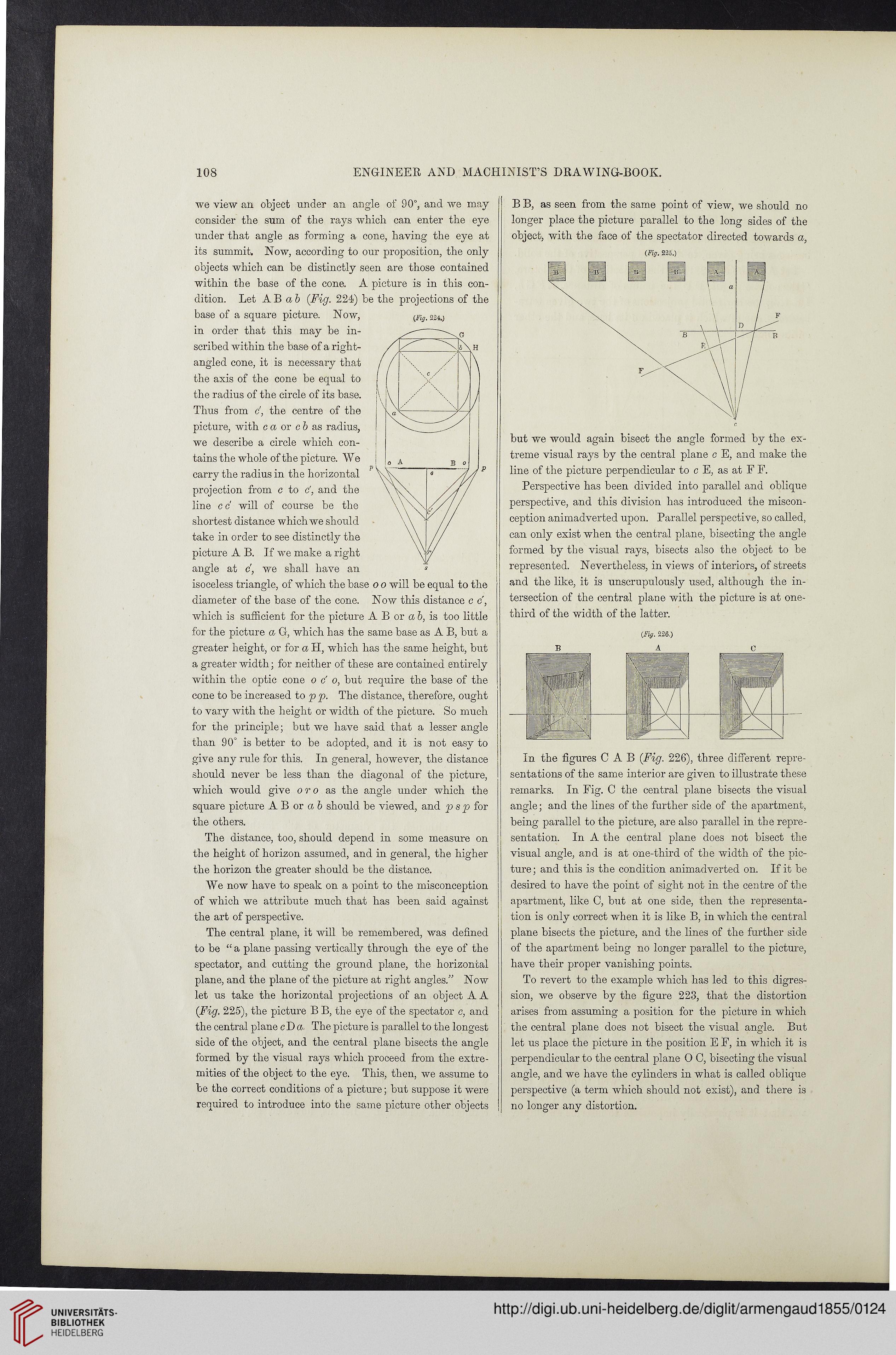
dition. Let A B a b (Fig. 224) be the projections of the
base of a square picture. Now,
in order that this may be in-
scribed within the base of a right-
angled cone, it is necessary that
the axis of the cone be equal to
the radius of the circle of its base.
Thus from d, the centre of the
picture, with c a or c b as radius,
we describe a circle which con-
tains the whole of the picture. We
carry the radius in the horizontal
projection from c to d, and the
line cc will of course be the
shortest distance which we should
take in order to see distinctly the
picture A B. If we make a right
angle at d, we shall have an
isoceless triangle, of which the base o o will be equal to the
diameter of the base of the cone. Now this distance c c,
which is sufficient for the picture A B or a b, is too little
for the picture a G, which has the same base as A B, but a
greater height, or for a H, which has the same height, but
a greater width; for neither of these are contained entirely
within the optic cone o d o, but require the base of the
cone to be increased to p p. The distance, therefore, ought
to vary with the height or width of the picture. So much
for the principle; but we have said that a lesser angle
than 90" is better to be adopted, and it is not easy to
give any rule for this. In general, however, the distance
should never be less than the diagonal of the picture,
which would give oro as the angle under which the
square picture A B or ab should be viewed, and p s p for
the others.
The distance, too, should depend in some measure on
the height of horizon assumed, and in general, the higher
the horizon the greater should be the distance.
We now have to speak on a point to the misconception
of which we attribute much that has been said against
the art of perspective.
The central plane, it will be remembered, was defined
to be “ a plane passing vertically through the eye of the
spectator, and cutting the ground plane, the horizontal
plane, and the plane of the picture at right angles/' Now
let us take the horizontal projections of an object A A
(Fig. 225), the picture B B, the eye of the spectator c, and
the central plane cDa. The picture is parallel to the longest
side of the object, and the central plane bisects the angle
formed by the visual rays which proceed from the extre-
mities of the object to the eye. This, then, we assume to
be the correct conditions of a picture; but suppose it were
required to introduce into the same picture other objects
{Fig. 224.J
B B, as seen from the same point of view, we should no
longer place the picture parallel to the long sides of the
object, with the face of the spectator directed towards a,
{Fig. 225.)
treme visual rays by the central plane c E, and make the
line of the picture perpendicular to c E, as at F F.
Perspective has been divided into parallel and oblique
perspective, and this division has introduced the miscon-
ception animadverted upon. Parallel perspective, so called,
can only exist when the central plane, bisecting the angle
formed by the visual rays, bisects also the object to be
represented. Nevertheless, in views of interiors, of streets
and the like, it is unscrupulously used, although the in-
tersection of the central plane with the picture is at one-
third of the width of the latter.
{Fig. 226.)
In the figures CAB (Fig. 226), three different repre-
sentations of the same interior are given to illustrate these
remarks. In Fig. C the central plane bisects the visual
angle; and the lines of the further side of the apartment,
being parallel to the picture, are also parallel in the repre-
sentation. In A the central plane does not bisect the
visual angle, and is at one-third of the width of the pic-
ture ; and this is the condition animadverted on. If it be
desired to have the point of sight not in the centre of the
apartment, like C, but at one side, then the representa-
tion is only correct when it is like B, in which the central
plane bisects the picture, and the lines of the further side
of the apartment being no longer parallel to the picture,
have their proper vanishing points.
To revert to the example which has led to this digres-
sion, we observe by the figure 223, that the distortion
arises from assuming a position for the picture in which
the central plane does not bisect the visual angle. But
let us place the picture in the position E F, in which it is
perpendicular to the central plane O C, bisecting the visual
angle, and we have the cylinders in what is called oblique
perspective (a term which should not exist), and there is
no longer any distortion.
ENGINEER AND MACHINIST’S DRAWING-BOOK.
we view an object under an angle of 90°, and we may
consider the sum of the rays which can enter the eye
under that angle as forming a cone, having the eye at
its summit. Now, according to our proposition, the only
objects which can be distinctly seen are those contained
within the base of the cone. A picture is in this con-
dition. Let A B a b (Fig. 224) be the projections of the
base of a square picture. Now,
in order that this may be in-
scribed within the base of a right-
angled cone, it is necessary that
the axis of the cone be equal to
the radius of the circle of its base.
Thus from d, the centre of the
picture, with c a or c b as radius,
we describe a circle which con-
tains the whole of the picture. We
carry the radius in the horizontal
projection from c to d, and the
line cc will of course be the
shortest distance which we should
take in order to see distinctly the
picture A B. If we make a right
angle at d, we shall have an
isoceless triangle, of which the base o o will be equal to the
diameter of the base of the cone. Now this distance c c,
which is sufficient for the picture A B or a b, is too little
for the picture a G, which has the same base as A B, but a
greater height, or for a H, which has the same height, but
a greater width; for neither of these are contained entirely
within the optic cone o d o, but require the base of the
cone to be increased to p p. The distance, therefore, ought
to vary with the height or width of the picture. So much
for the principle; but we have said that a lesser angle
than 90" is better to be adopted, and it is not easy to
give any rule for this. In general, however, the distance
should never be less than the diagonal of the picture,
which would give oro as the angle under which the
square picture A B or ab should be viewed, and p s p for
the others.
The distance, too, should depend in some measure on
the height of horizon assumed, and in general, the higher
the horizon the greater should be the distance.
We now have to speak on a point to the misconception
of which we attribute much that has been said against
the art of perspective.
The central plane, it will be remembered, was defined
to be “ a plane passing vertically through the eye of the
spectator, and cutting the ground plane, the horizontal
plane, and the plane of the picture at right angles/' Now
let us take the horizontal projections of an object A A
(Fig. 225), the picture B B, the eye of the spectator c, and
the central plane cDa. The picture is parallel to the longest
side of the object, and the central plane bisects the angle
formed by the visual rays which proceed from the extre-
mities of the object to the eye. This, then, we assume to
be the correct conditions of a picture; but suppose it were
required to introduce into the same picture other objects
{Fig. 224.J
B B, as seen from the same point of view, we should no
longer place the picture parallel to the long sides of the
object, with the face of the spectator directed towards a,
{Fig. 225.)
treme visual rays by the central plane c E, and make the
line of the picture perpendicular to c E, as at F F.
Perspective has been divided into parallel and oblique
perspective, and this division has introduced the miscon-
ception animadverted upon. Parallel perspective, so called,
can only exist when the central plane, bisecting the angle
formed by the visual rays, bisects also the object to be
represented. Nevertheless, in views of interiors, of streets
and the like, it is unscrupulously used, although the in-
tersection of the central plane with the picture is at one-
third of the width of the latter.
{Fig. 226.)
In the figures CAB (Fig. 226), three different repre-
sentations of the same interior are given to illustrate these
remarks. In Fig. C the central plane bisects the visual
angle; and the lines of the further side of the apartment,
being parallel to the picture, are also parallel in the repre-
sentation. In A the central plane does not bisect the
visual angle, and is at one-third of the width of the pic-
ture ; and this is the condition animadverted on. If it be
desired to have the point of sight not in the centre of the
apartment, like C, but at one side, then the representa-
tion is only correct when it is like B, in which the central
plane bisects the picture, and the lines of the further side
of the apartment being no longer parallel to the picture,
have their proper vanishing points.
To revert to the example which has led to this digres-
sion, we observe by the figure 223, that the distortion
arises from assuming a position for the picture in which
the central plane does not bisect the visual angle. But
let us place the picture in the position E F, in which it is
perpendicular to the central plane O C, bisecting the visual
angle, and we have the cylinders in what is called oblique
perspective (a term which should not exist), and there is
no longer any distortion.