PERSPECTIVE.
109
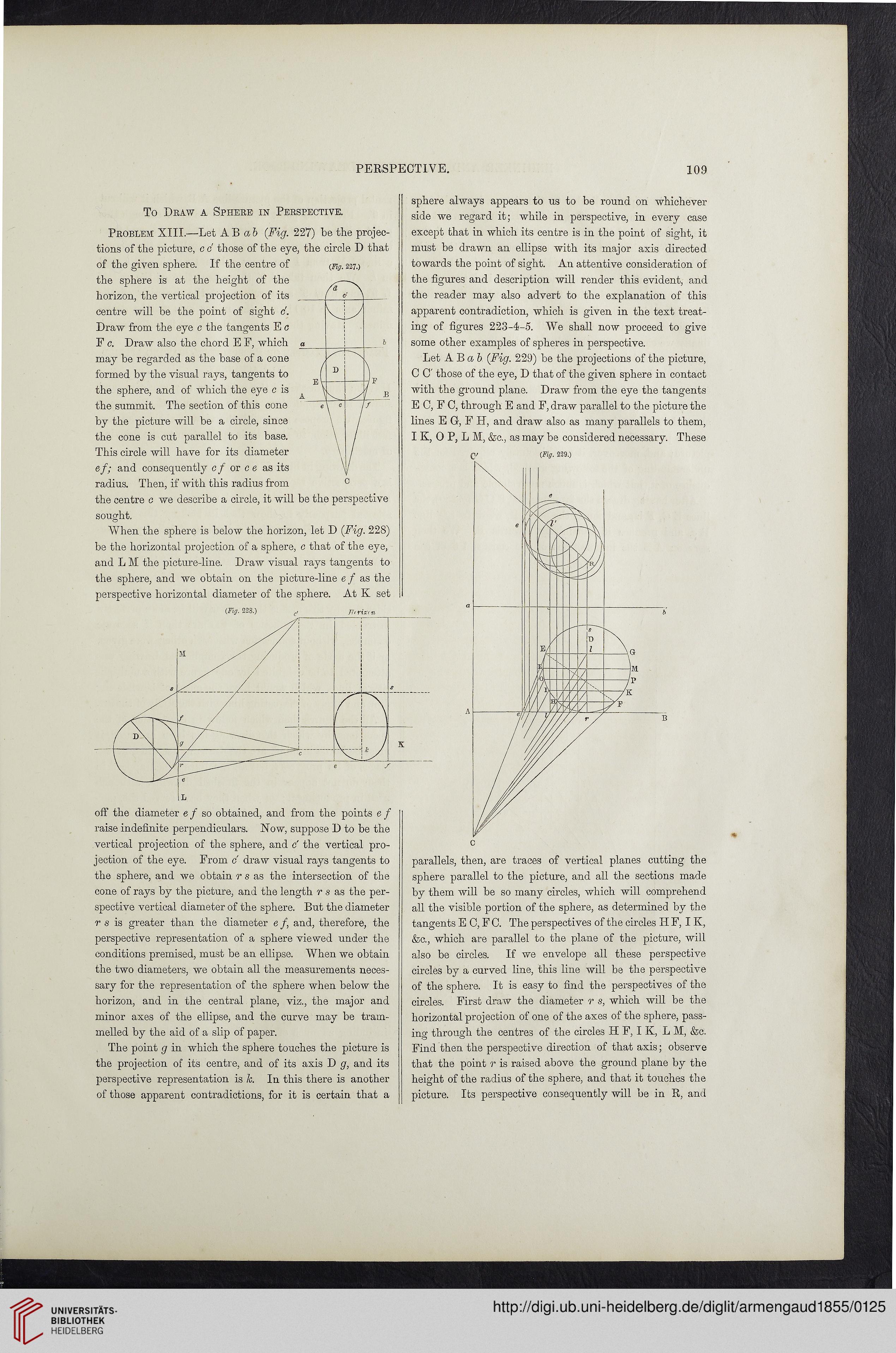
To Draw a Sphere in Perspective.
Problem XIII.—Let AB ab (Fig. 227) be the projec-
tions of the picture, c c those of the eye, the circle D that
of the given sphere. If the centre of
the sphere is at the height of the
horizon, the vertical projection of its
centre will be the point of sight 6.
Draw from the eye c the tangents E c
F c. Draw also the chord E F, which
may be regarded as the base of a cone
formed by the visual rays, tangents to
the sphere, and of which the eye c is
the summit. The section of this cone
by the picture will be a circle, since
the cone is cut parallel to its base.
This circle will have for its diameter
ef; and consequently of or c e as its
radius. Then, if with this radius from
the centre e we describe a circle, it will be the perspective
sought.
When the sphere is below the horizon, let D (Fig. 228)
be the horizontal projection of a sphere, c that of the eye,
and L M the picture-line. Draw visual rays tangents to
the sphere, and we obtain on the picture-line e f as the
perspective horizontal diameter of the sphere. At K set
(Fit7.228.) c, Jhrixtn
off the diameter ef so obtained, and from the points e f
raise indefinite perpendiculars. Now, suppose D to be the
vertical projection of the sphere, and c' the vertical pro-
jection of the eye. From c draw visual rays tangents to
the sphere, and we obtain r s as the intersection of the
cone of rays by the picture, and the length r s as the per-
spective vertical diameter of the sphere. But the diameter
r s is greater than the diameter e f, and, therefore, the
perspective representation of a sphere viewed under the
conditions premised, must be an ellipse. When we obtain
the two diameters, we obtain all the measurements neces-
sary for the representation of the sphere when below the
horizon, and in the central plane, viz., the major and
minor axes of the ellipse, and the curve may be tram-
melled by the aid of a slip of paper.
The point g in which the sphere touches the picture is
the projection of its centre, and of its axis D g, and its
perspective representation is 1c. In this there is another
of those apparent contradictions, for it is certain that a
sphere always appears to us to be round on whichever
side we regard it; while in perspective, in every case
except that in which its centre is in the point of sight, it
must be drawn an ellipse with its major axis directed
towards the point of sight. An attentive consideration of
the figures and description will render this evident, and
the reader may also advert to the explanation of this
apparent contradiction, which is given in the text treat-
ing of figures 223-4-5. We shall now proceed to give
some other examples of spheres in perspective.
Let A B ab (Fig. 229) be the projections of the picture,
C C' those of the eye, D that of the given sphere in contact
with the ground plane. Draw from the eye the tangents
E C, F C, through E and F, draw parallel to the picture the
lines E G, F H, and draw also as many parallels to them,
I K, 0 P, L M, &c., as may be considered necessary. These
fi/ (Fig. 229.)
parallels, then, are traces of vertical planes cutting the
sphere parallel to the picture, and all the sections made
by them will be so many circles, which will comprehend
all the visible portion of the sphere, as determined by the
tangents E C, F C. The perspectives of the circles IIF, IK,
&c., which are parallel to the plane of the picture, will
also be circles. If we envelope all these perspective
circles by a curved line, this line will be the perspective
of the sphere. It is easy to find the perspectives of the
circles. First draw the diameter r s, which will be the
horizontal projection of one of the axes of the sphere, pass-
ing through the centres of the circles H F, I K, L M, &c.
Find then the perspective direction of that axis; observe
that the point r is raised above the ground plane by the
height of the radius of the sphere, and that it touches the
picture. Its perspective consequently will be in R, and
(Fig. 227.)
109
To Draw a Sphere in Perspective.
Problem XIII.—Let AB ab (Fig. 227) be the projec-
tions of the picture, c c those of the eye, the circle D that
of the given sphere. If the centre of
the sphere is at the height of the
horizon, the vertical projection of its
centre will be the point of sight 6.
Draw from the eye c the tangents E c
F c. Draw also the chord E F, which
may be regarded as the base of a cone
formed by the visual rays, tangents to
the sphere, and of which the eye c is
the summit. The section of this cone
by the picture will be a circle, since
the cone is cut parallel to its base.
This circle will have for its diameter
ef; and consequently of or c e as its
radius. Then, if with this radius from
the centre e we describe a circle, it will be the perspective
sought.
When the sphere is below the horizon, let D (Fig. 228)
be the horizontal projection of a sphere, c that of the eye,
and L M the picture-line. Draw visual rays tangents to
the sphere, and we obtain on the picture-line e f as the
perspective horizontal diameter of the sphere. At K set
(Fit7.228.) c, Jhrixtn
off the diameter ef so obtained, and from the points e f
raise indefinite perpendiculars. Now, suppose D to be the
vertical projection of the sphere, and c' the vertical pro-
jection of the eye. From c draw visual rays tangents to
the sphere, and we obtain r s as the intersection of the
cone of rays by the picture, and the length r s as the per-
spective vertical diameter of the sphere. But the diameter
r s is greater than the diameter e f, and, therefore, the
perspective representation of a sphere viewed under the
conditions premised, must be an ellipse. When we obtain
the two diameters, we obtain all the measurements neces-
sary for the representation of the sphere when below the
horizon, and in the central plane, viz., the major and
minor axes of the ellipse, and the curve may be tram-
melled by the aid of a slip of paper.
The point g in which the sphere touches the picture is
the projection of its centre, and of its axis D g, and its
perspective representation is 1c. In this there is another
of those apparent contradictions, for it is certain that a
sphere always appears to us to be round on whichever
side we regard it; while in perspective, in every case
except that in which its centre is in the point of sight, it
must be drawn an ellipse with its major axis directed
towards the point of sight. An attentive consideration of
the figures and description will render this evident, and
the reader may also advert to the explanation of this
apparent contradiction, which is given in the text treat-
ing of figures 223-4-5. We shall now proceed to give
some other examples of spheres in perspective.
Let A B ab (Fig. 229) be the projections of the picture,
C C' those of the eye, D that of the given sphere in contact
with the ground plane. Draw from the eye the tangents
E C, F C, through E and F, draw parallel to the picture the
lines E G, F H, and draw also as many parallels to them,
I K, 0 P, L M, &c., as may be considered necessary. These
fi/ (Fig. 229.)
parallels, then, are traces of vertical planes cutting the
sphere parallel to the picture, and all the sections made
by them will be so many circles, which will comprehend
all the visible portion of the sphere, as determined by the
tangents E C, F C. The perspectives of the circles IIF, IK,
&c., which are parallel to the plane of the picture, will
also be circles. If we envelope all these perspective
circles by a curved line, this line will be the perspective
of the sphere. It is easy to find the perspectives of the
circles. First draw the diameter r s, which will be the
horizontal projection of one of the axes of the sphere, pass-
ing through the centres of the circles H F, I K, L M, &c.
Find then the perspective direction of that axis; observe
that the point r is raised above the ground plane by the
height of the radius of the sphere, and that it touches the
picture. Its perspective consequently will be in R, and
(Fig. 227.)