PERSPECTIVE.
113
draw K L at right angles to the diagonal A 1), and K L
is the trace of the plane of projection.
If to this line we draw through the points E C F B of
the cube, lines parallel to the diagonal A D, and therefore
perpendicular to K L, we find that the projection c D of
the edge of the cube A C, is equal to the projections of
the diagonals A B, CD, of the top and bottom surfaces of
the cube, and we know that the projection of the other
diagonal C' F' of the top (No. 2), and the projection of
one diagonal on each side of the cube, will be equal to
the original line, as they lie in planes parallel to the plane
of projection.
Produce A D indefinitely, and at any point of it A"
(No. 3), with the radius D c or D b describe a circle. Draw
its other diameter B" c", and produce the lines E e F/
through its circumference; join the points c E' F B'/' e c",
to complete the hexagon, then join A" F', A"/', and we
have the isometrical projection of a cube, one of the sides
of which is r B s D.
The lines drawn from the plan above (No. 4), show
that the projection F'/' of the diagonal C D is of the
same size as the original, and the triangle c" F' /', which
is the projection of the section of the cube by a plane C F'
(No. 1), parallel to K L, the plane of projection shows
that the projection of one diagonal on each of the sides of
the cube must also be of the same size as the original.
The relations of the lines of the projection to the ori-
ginal lines, are as follows :—The lines c" F', F' /', and/' c",
and all lines parallel to them, are equal to their original
lines.
The isometrical axes and isometrical lines are to the
original lines as ’8164 to 1.
The diagonals A" B", A" E', and A C' are to their ori-
ginals as -5773 to 1 ; or otherwise, calling the minor axis
unity, then the isometrical lines are 1’41421, and the
major axis equal to the original, is 1’73205, their ratio
being as VI, vws-
But in practice it is not necessary to find these lengths
by computation; it is much more simple and easy to con-
struct scales having the proper relation to each other, as
we shall proceed to show in Fig. 233.
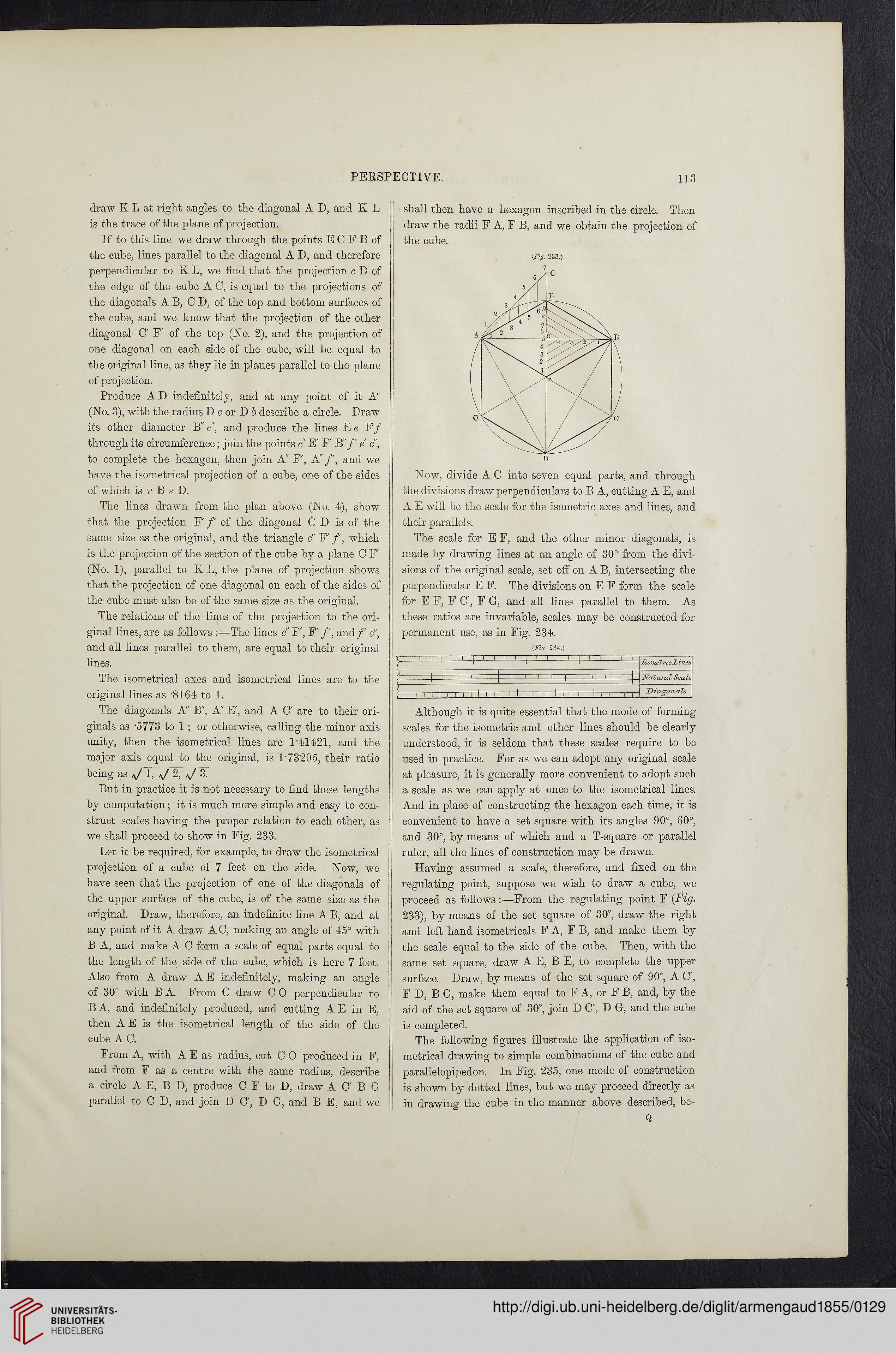
Let it be required, for example, to draw the isometrical
projection of a cube of 7 feet on the side. Now, we
have seen that the projection of one of the diagonals of j
the upper surface of the cube, is of the same size as the |
original. Draw, therefore, an indefinite line A B, and at
any point of it A draw A C, making an angle of 45° with
B A, and make A C form a scale of equal parts equal to
the length of the side of the cube, which is here 7 feet.
Also from A draw A E indefinitely, making an angle
of 30° with B A. From C draw C 0 perpendicular to
B A, and indefinitely produced, and cutting A E in E,
then A E is the isometrical length of the side of the
cube A C.
From A, with A E as radius, cut C 0 produced in F,
and from F as a centre with the same radius, describe
a circle A E, B D, produce C F to D, draw A C' B G
parallel to C D, and join D C', D G, and B E, and we
shall then have a hexagon inscribed in the circle. Then
draw the radii F A, F B, and we obtain the projection of
the cube.
(Fig. 2SS.)
N ow, divide A C into seven equal parts, and through
the divisions draw perpendiculars to B A, cutting A E, and
A E will be the scale for the isometric axes and lines, and
their parallels.
The scale for E F, and the other minor diagonals, is
made by drawing lines at an angle of 30° from the divi-
sions of the original scale, set off on A B, intersecting the
perpendicular E F. The divisions on E F form the scale
for E F, F C', F G, and all lines parallel to them. As
these ratios are invariable, scales may be constructed for
permanent use, as in Fig. 234.
(Fig, 234.)
\_I 1 "TT i_1_1_!_1_l_1_!_1_1_I_!_I 1 1 1
Isometric I ines
>-1 i 1 1
NotZizraL Scale
s 1 11
-1-!-1-1-1-1-1-1-1-
$---;-
Diagonals
)- ill! 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 I 1
Although it is quite essential that the mode of forming-
scales for the isometric and other lines should be clearly
understood, it is seldom that these scales require to be
used in practice. For as we can adopt any original scale
at pleasure, it is generally more convenient to adopt such
a scale as we can apply at once to the isometrical lines.
And in place of constructing the hexagon each time, it is
convenient to have a set square with its angles 90°, 60°,
and 30°, by means of which and a T-square or parallel
ruler, all the lines of construction may be drawn.
Having assumed a scale, therefore, and fixed on the
regulating point, suppose we wish to draw a cube, we
proceed as follows:—From the regulating point F (Fig.
233), by means of the set square of 30°, draw the right
and left hand isometricals FA, F B, and make them by
the scale equal to the side of the cube. Then, with the
same set square, draw A E, B E, to complete the upper
surface. Draw, by means of the set square of 90°, A C',
F D, B G, make them equal to F A, or F B, and, by the
aid of the set square of 30°, join D C', D G, and the cube
is completed.
The following figures illustrate the application of iso-
metrical drawing to simple combinations of the cube and
parallelopipedon. In Fig. 235, one mode of construction
is shown by dotted lines, but we may proceed directly as
in drawing the cube in the manner above described, be-
113
draw K L at right angles to the diagonal A 1), and K L
is the trace of the plane of projection.
If to this line we draw through the points E C F B of
the cube, lines parallel to the diagonal A D, and therefore
perpendicular to K L, we find that the projection c D of
the edge of the cube A C, is equal to the projections of
the diagonals A B, CD, of the top and bottom surfaces of
the cube, and we know that the projection of the other
diagonal C' F' of the top (No. 2), and the projection of
one diagonal on each side of the cube, will be equal to
the original line, as they lie in planes parallel to the plane
of projection.
Produce A D indefinitely, and at any point of it A"
(No. 3), with the radius D c or D b describe a circle. Draw
its other diameter B" c", and produce the lines E e F/
through its circumference; join the points c E' F B'/' e c",
to complete the hexagon, then join A" F', A"/', and we
have the isometrical projection of a cube, one of the sides
of which is r B s D.
The lines drawn from the plan above (No. 4), show
that the projection F'/' of the diagonal C D is of the
same size as the original, and the triangle c" F' /', which
is the projection of the section of the cube by a plane C F'
(No. 1), parallel to K L, the plane of projection shows
that the projection of one diagonal on each of the sides of
the cube must also be of the same size as the original.
The relations of the lines of the projection to the ori-
ginal lines, are as follows :—The lines c" F', F' /', and/' c",
and all lines parallel to them, are equal to their original
lines.
The isometrical axes and isometrical lines are to the
original lines as ’8164 to 1.
The diagonals A" B", A" E', and A C' are to their ori-
ginals as -5773 to 1 ; or otherwise, calling the minor axis
unity, then the isometrical lines are 1’41421, and the
major axis equal to the original, is 1’73205, their ratio
being as VI, vws-
But in practice it is not necessary to find these lengths
by computation; it is much more simple and easy to con-
struct scales having the proper relation to each other, as
we shall proceed to show in Fig. 233.
Let it be required, for example, to draw the isometrical
projection of a cube of 7 feet on the side. Now, we
have seen that the projection of one of the diagonals of j
the upper surface of the cube, is of the same size as the |
original. Draw, therefore, an indefinite line A B, and at
any point of it A draw A C, making an angle of 45° with
B A, and make A C form a scale of equal parts equal to
the length of the side of the cube, which is here 7 feet.
Also from A draw A E indefinitely, making an angle
of 30° with B A. From C draw C 0 perpendicular to
B A, and indefinitely produced, and cutting A E in E,
then A E is the isometrical length of the side of the
cube A C.
From A, with A E as radius, cut C 0 produced in F,
and from F as a centre with the same radius, describe
a circle A E, B D, produce C F to D, draw A C' B G
parallel to C D, and join D C', D G, and B E, and we
shall then have a hexagon inscribed in the circle. Then
draw the radii F A, F B, and we obtain the projection of
the cube.
(Fig. 2SS.)
N ow, divide A C into seven equal parts, and through
the divisions draw perpendiculars to B A, cutting A E, and
A E will be the scale for the isometric axes and lines, and
their parallels.
The scale for E F, and the other minor diagonals, is
made by drawing lines at an angle of 30° from the divi-
sions of the original scale, set off on A B, intersecting the
perpendicular E F. The divisions on E F form the scale
for E F, F C', F G, and all lines parallel to them. As
these ratios are invariable, scales may be constructed for
permanent use, as in Fig. 234.
(Fig, 234.)
\_I 1 "TT i_1_1_!_1_l_1_!_1_1_I_!_I 1 1 1
Isometric I ines
>-1 i 1 1
NotZizraL Scale
s 1 11
-1-!-1-1-1-1-1-1-1-
$---;-
Diagonals
)- ill! 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 I 1
Although it is quite essential that the mode of forming-
scales for the isometric and other lines should be clearly
understood, it is seldom that these scales require to be
used in practice. For as we can adopt any original scale
at pleasure, it is generally more convenient to adopt such
a scale as we can apply at once to the isometrical lines.
And in place of constructing the hexagon each time, it is
convenient to have a set square with its angles 90°, 60°,
and 30°, by means of which and a T-square or parallel
ruler, all the lines of construction may be drawn.
Having assumed a scale, therefore, and fixed on the
regulating point, suppose we wish to draw a cube, we
proceed as follows:—From the regulating point F (Fig.
233), by means of the set square of 30°, draw the right
and left hand isometricals FA, F B, and make them by
the scale equal to the side of the cube. Then, with the
same set square, draw A E, B E, to complete the upper
surface. Draw, by means of the set square of 90°, A C',
F D, B G, make them equal to F A, or F B, and, by the
aid of the set square of 30°, join D C', D G, and the cube
is completed.
The following figures illustrate the application of iso-
metrical drawing to simple combinations of the cube and
parallelopipedon. In Fig. 235, one mode of construction
is shown by dotted lines, but we may proceed directly as
in drawing the cube in the manner above described, be-