34
ENGINEER AND MACHINIST’S DRAWING BOOK.
the square by a draw-point or pencil D. In nliis way the
curve will be described as required.
SECTION VI.
Architectural Elements.
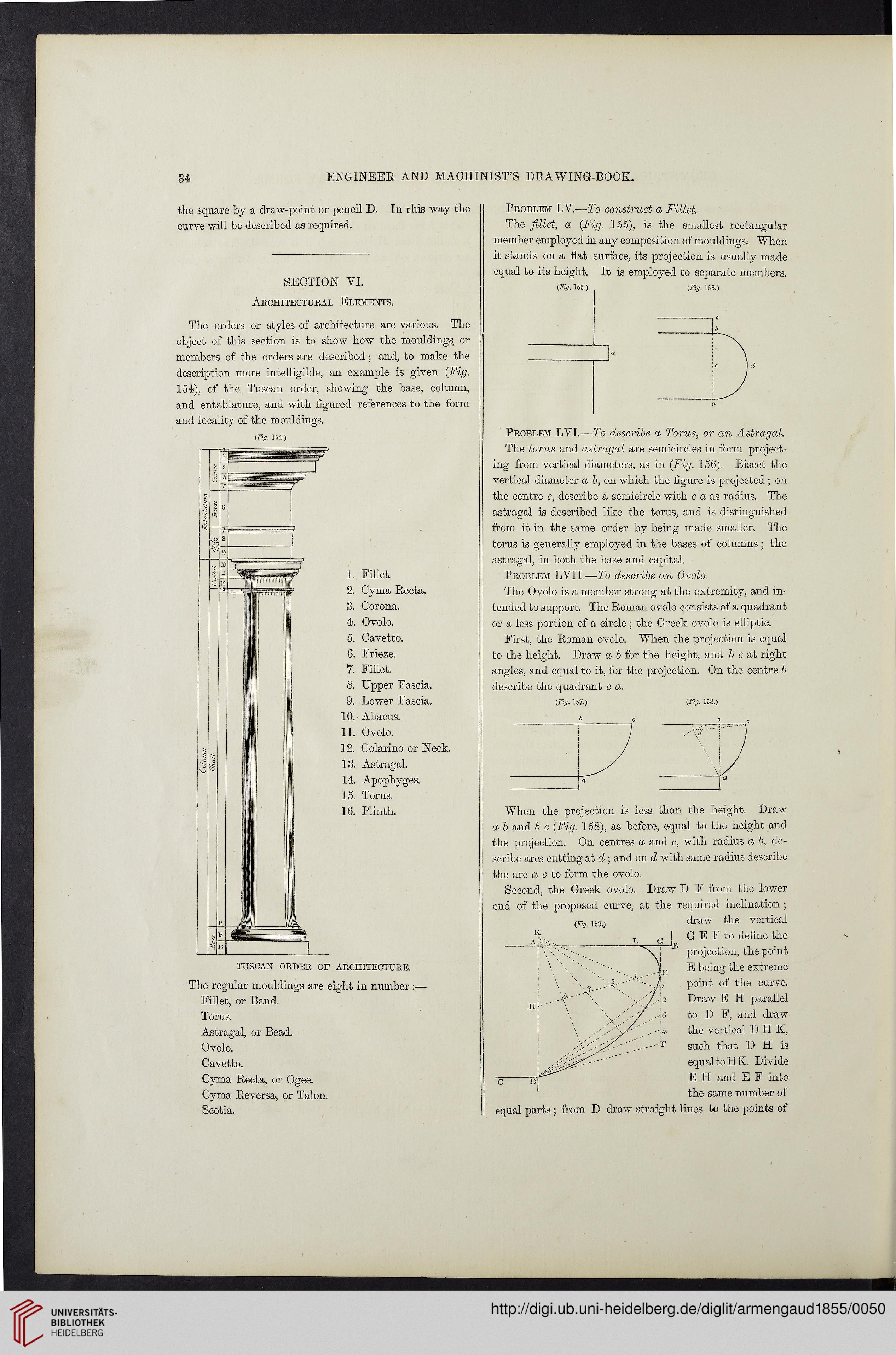
The orders or styles of architecture are various. The
object of this section is to show how the mouldings or
members of the orders are described; and, to make the
description more intelligible, an example is given {Fig.
154), of the Tuscan order, showing the base, column,
and entablature, and with figured references to the form
and locality of the mouldings.
(Fig. 154.)
TUSCAN ORDER OF
1. Fillet.
2. Cyma Recta.
3. Corona.
4. Ovolo.
5. Cavetto.
6. Frieze.
7. Fillet.
8. Upper Fascia.
9. Lower Fascia.
10. Abacus.
11. Ovolo.
12. Colarino or Neck.
13. Astragal.
14. Apophyges.
15. Torus.
16. Plinth.
ARCHITECTURE.
The regular mouldings are eight in number :—
Fillet, or Band.
Torus.
Astragal, or Bead,
Ovolo.
Cavetto.
Cyma Recta, or Ogee.
Cyma Re versa, or Talon.
Scotia.
Problem LV.—To construct a Fillet
The fillet, a {Fig. 155), is the smallest rectangular
member employed in any composition of mouldings. When
it stands on a flat surface, its projection is usually made
equal to its height. It is employed to separate members.
(Fig. 155.) (Fig. 156.)
Problem LYI.—To describe a Torus, or an Astragal.
The torus and astragal are semicircles in form project-
ing from vertical diameters, as in {Fig. 156). Bisect the
vertical diameter a b, on which the figure is projected ; on
the centre c, describe a semicircle with c a as radius. The
astragal is described like the torus, and is distinguished
from it in the same order by being made smaller. The
torus is generally employed in the bases of columns; the
astragal, in both the base and capital.
Problem LVII.—To describe an Ovolo.
The Ovolo is a member strong at the extremity, and in-
tended to support. The Roman ovolo consists of a quadrant
or a less portion of a circle; the Greek ovolo is elliptic.
First, the Roman ovolo. When the projection is equal
to the height. Draw a b for the height, and be at right
angles, and equal to it, for the projection. On the centre b
describe the quadrant c a.
(Fig. 1570 (fig- 158.)
(Fig. 159.)
When the projection is less than the height. Draw
a b and b c {Fig. 158), as before, equal to the height and
the projection. On centres a and c, with radius a b, de-
scribe arcs cutting at d; and on d with same radius describe
the arc a c to form the ovolo.
Second, the Greek ovolo. Draw D F from the lower
end of the proposed curve, at the required inclination ;
draw the vertical
G E F to define the
projection, the point
E being the extreme
point of the curve.
Draw E H parallel
to D F, and draw
the vertical D H K,
such that D H is
equal to HK. Divide
E H and E F into
the same number of
equal parts; from D draw straight lines to the points of
ENGINEER AND MACHINIST’S DRAWING BOOK.
the square by a draw-point or pencil D. In nliis way the
curve will be described as required.
SECTION VI.
Architectural Elements.
The orders or styles of architecture are various. The
object of this section is to show how the mouldings or
members of the orders are described; and, to make the
description more intelligible, an example is given {Fig.
154), of the Tuscan order, showing the base, column,
and entablature, and with figured references to the form
and locality of the mouldings.
(Fig. 154.)
TUSCAN ORDER OF
1. Fillet.
2. Cyma Recta.
3. Corona.
4. Ovolo.
5. Cavetto.
6. Frieze.
7. Fillet.
8. Upper Fascia.
9. Lower Fascia.
10. Abacus.
11. Ovolo.
12. Colarino or Neck.
13. Astragal.
14. Apophyges.
15. Torus.
16. Plinth.
ARCHITECTURE.
The regular mouldings are eight in number :—
Fillet, or Band.
Torus.
Astragal, or Bead,
Ovolo.
Cavetto.
Cyma Recta, or Ogee.
Cyma Re versa, or Talon.
Scotia.
Problem LV.—To construct a Fillet
The fillet, a {Fig. 155), is the smallest rectangular
member employed in any composition of mouldings. When
it stands on a flat surface, its projection is usually made
equal to its height. It is employed to separate members.
(Fig. 155.) (Fig. 156.)
Problem LYI.—To describe a Torus, or an Astragal.
The torus and astragal are semicircles in form project-
ing from vertical diameters, as in {Fig. 156). Bisect the
vertical diameter a b, on which the figure is projected ; on
the centre c, describe a semicircle with c a as radius. The
astragal is described like the torus, and is distinguished
from it in the same order by being made smaller. The
torus is generally employed in the bases of columns; the
astragal, in both the base and capital.
Problem LVII.—To describe an Ovolo.
The Ovolo is a member strong at the extremity, and in-
tended to support. The Roman ovolo consists of a quadrant
or a less portion of a circle; the Greek ovolo is elliptic.
First, the Roman ovolo. When the projection is equal
to the height. Draw a b for the height, and be at right
angles, and equal to it, for the projection. On the centre b
describe the quadrant c a.
(Fig. 1570 (fig- 158.)
(Fig. 159.)
When the projection is less than the height. Draw
a b and b c {Fig. 158), as before, equal to the height and
the projection. On centres a and c, with radius a b, de-
scribe arcs cutting at d; and on d with same radius describe
the arc a c to form the ovolo.
Second, the Greek ovolo. Draw D F from the lower
end of the proposed curve, at the required inclination ;
draw the vertical
G E F to define the
projection, the point
E being the extreme
point of the curve.
Draw E H parallel
to D F, and draw
the vertical D H K,
such that D H is
equal to HK. Divide
E H and E F into
the same number of
equal parts; from D draw straight lines to the points of