102
THE ENGINEER AND MACHINIST’S DRAWING-BOOK.
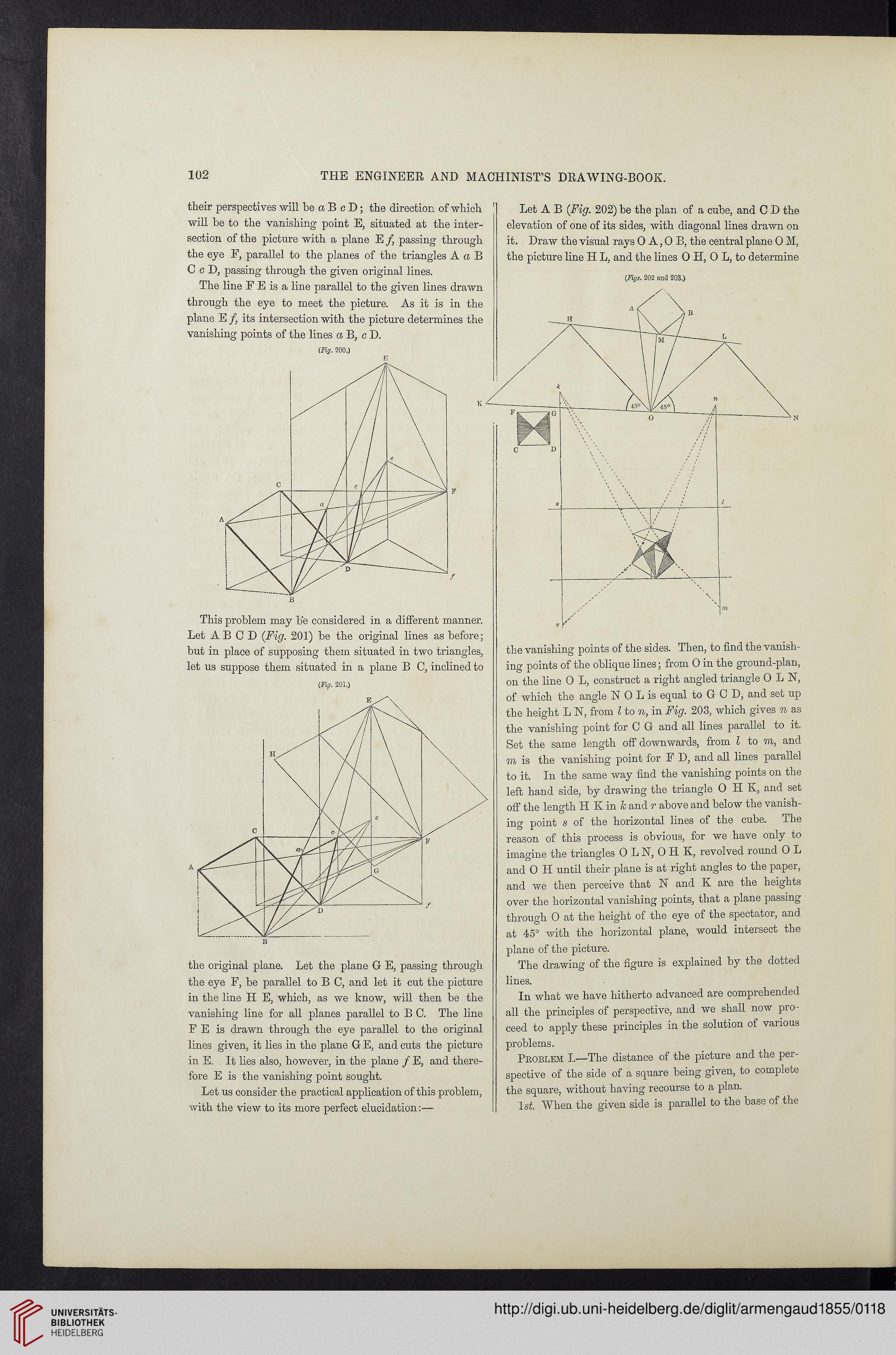
their perspectives will be a B c D; the direction of which
will be to the vanishing point E, situated at the inter-
section of the picture with a plane E f, passing through
the eye F, parallel to the planes of the triangles A a B
C c D, passing through the given original lines.
The line F E is a line parallel to the given lines drawn
through the eye to meet the picture. As it is in the
plane E /. its intersection with the picture determines the
vanishing points of the lines a B, cD.
[Fig. 200.)
E
This problem may be considered in a different manner.
Let AB C D (Fig. 201) be the original lines as before;
but in place of supposing them situated in two triangles,
let us suppose them situated in a plane B C, inclined to
[Fig. 201.)
the original plane. Let the plane G E, passing through
the eye F, be parallel to B C, and let it cut the picture
in the line H E, which, as we know, will then be the
vanishing line for all planes parallel to B C. The line
F E is drawn through the eye parallel to the original
lines given, it lies in the plane G E, and cuts the picture
in E. It lies also, however, in the plane / E, and there-
fore E is the vanishing point sought.
Let us consider the practical application of this problem,
with the view to its more perfect elucidation :•—
Let A B (Fig. 202) be the plan of a cube, and C D the
elevation of one of its sides, with diagonal lines drawn on
it. Draw the visual rays O A, O B, the central plane O M,
the picture line H L, and the lines O H, O L, to determine
[Figs. 202 and 203,)
/\
' m
f/
the vanishing points of the sides. Then, to find the vanish-
ing points of the oblique lines; from O in the ground-plan,
on the line O L, construct a right angled triangle O L N,
of which the angle N O L is equal to G C D, and set up
the height L N, from l to n, in Fig. 203, which gives n as
the vanishing point for C G and all lines parallel to it.
Set the same length off downwards, from l to to, and
to is the vanishing point for F D, and all lines parallel
to it. In the same way find the vanishing points on the
left hand side, by drawing the triangle O H K, and set
off the length H K in Jc and r above and below the vanish-
ing point s of the horizontal lines of the cube. The
reason of this process is obvious, for we have only to
imagine the triangles OLN,OHK, revolved round O L
and O H until their plane is at right angles to the paper,
and we then perceive that N and K are the heights
over the horizontal vanishing points, that a plane passing
through O at the height of the eye of the spectator, and
at 45° with the horizontal plane, would intersect the
plane of the picture.
The drawing of the figure is esplained by the dotted
lines.
In what we have hitherto advanced are comprehended
all the principles of perspective, and we shall now pro-
ceed to apply these principles in the solution of various
problems.
Problem I.—The distance of the picture and the per-
spective of the side of a square being given, to complete
the square, without having recourse to a plan.
1st. When the given side is parallel to the base of the
THE ENGINEER AND MACHINIST’S DRAWING-BOOK.
their perspectives will be a B c D; the direction of which
will be to the vanishing point E, situated at the inter-
section of the picture with a plane E f, passing through
the eye F, parallel to the planes of the triangles A a B
C c D, passing through the given original lines.
The line F E is a line parallel to the given lines drawn
through the eye to meet the picture. As it is in the
plane E /. its intersection with the picture determines the
vanishing points of the lines a B, cD.
[Fig. 200.)
E
This problem may be considered in a different manner.
Let AB C D (Fig. 201) be the original lines as before;
but in place of supposing them situated in two triangles,
let us suppose them situated in a plane B C, inclined to
[Fig. 201.)
the original plane. Let the plane G E, passing through
the eye F, be parallel to B C, and let it cut the picture
in the line H E, which, as we know, will then be the
vanishing line for all planes parallel to B C. The line
F E is drawn through the eye parallel to the original
lines given, it lies in the plane G E, and cuts the picture
in E. It lies also, however, in the plane / E, and there-
fore E is the vanishing point sought.
Let us consider the practical application of this problem,
with the view to its more perfect elucidation :•—
Let A B (Fig. 202) be the plan of a cube, and C D the
elevation of one of its sides, with diagonal lines drawn on
it. Draw the visual rays O A, O B, the central plane O M,
the picture line H L, and the lines O H, O L, to determine
[Figs. 202 and 203,)
/\
' m
f/
the vanishing points of the sides. Then, to find the vanish-
ing points of the oblique lines; from O in the ground-plan,
on the line O L, construct a right angled triangle O L N,
of which the angle N O L is equal to G C D, and set up
the height L N, from l to n, in Fig. 203, which gives n as
the vanishing point for C G and all lines parallel to it.
Set the same length off downwards, from l to to, and
to is the vanishing point for F D, and all lines parallel
to it. In the same way find the vanishing points on the
left hand side, by drawing the triangle O H K, and set
off the length H K in Jc and r above and below the vanish-
ing point s of the horizontal lines of the cube. The
reason of this process is obvious, for we have only to
imagine the triangles OLN,OHK, revolved round O L
and O H until their plane is at right angles to the paper,
and we then perceive that N and K are the heights
over the horizontal vanishing points, that a plane passing
through O at the height of the eye of the spectator, and
at 45° with the horizontal plane, would intersect the
plane of the picture.
The drawing of the figure is esplained by the dotted
lines.
In what we have hitherto advanced are comprehended
all the principles of perspective, and we shall now pro-
ceed to apply these principles in the solution of various
problems.
Problem I.—The distance of the picture and the per-
spective of the side of a square being given, to complete
the square, without having recourse to a plan.
1st. When the given side is parallel to the base of the